NCERT Solutions Class 7 Mathematics
Chapter – 12 (Algebraic Expressions)
The NCERT Solutions in English Language for Class 7 Mathematics Chapter – 12 Algebraic Expressions Exercise 12.1 has been provided here to help the students in solving the questions from this exercise.
Chapter : 12 Algebraic Expressions
- NCERT Solution Class 7 Maths Exercise – 12.2
- NCERT Solution Class 7 Maths Exercise – 12.3
- NCERT Solution Class 7 Maths Exercise – 12.4
Exercise – 12.1
1. Get the algebraic expressions in the following cases using variables, constants and arithmetic operations.
(i) Subtraction of z from y.
(ii) One half of the sum of numbers x and y.
(iii) The number z multiplied by itself.
(iv) One-fourth of the product of numbers p and q.
(v) Numbers x and y both squared and added.
(vi) Number 5 added to three times the product of number m and n.
(vii) Product of numbers y and 2 subtracted from 10.
(viii) Sum of numbers a and b subtracted from their product.
Solution –
(i) Subtraction of z from y
⇒ y – z
(ii) One half of the sum of numbers x and y
⇒
(iii) The number 2 multiplied by itself.
⇒ z × z = z2
(iv) One-fourth of the product of numbers p and q
⇒
(v) Numbers x and y both squared and added
⇒ x2 + y2
(vi) Number 5 added to three times the product of number m and n
⇒ 3mn + 5
(vii) Product of numbers y and z subtracted from 10
⇒ 10 – yz
(viii) Sum of numbers a and 6 subtracted from their product
⇒ Sum = a + b,
Product = ab
∴ Required expression
= ab – (a + b)
= ab – a- b
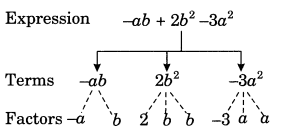
2. (i) Identify the terms and their factors in the following expressions
Show the terms and factors by tree diagrams.
(a) x – 3
(b) 1 + x + x2
(c) y – y3
(d) 5xy2 + 7x2y
(e) -ab + 2b2 – 3a2
Solution –
(a) x – 3

(b) 1 + x + x2
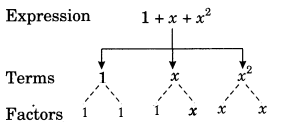
(c) y – y3
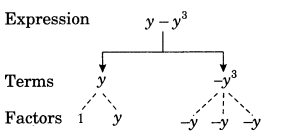
(d) 5xy2 + 7x2y
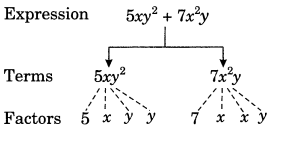
(e) – ab + 2b2 – 3a2
(ii) Identify terms and factors in the expressions given below:
(a) – 4x + 5
(b) – 4x + 5y
(c) 5y + 3y2
(d) xy + 2x2y2
(e) pq + q
(f) 1.2 ab – 2.4 b + 3.6 a
(g) x +
(h) 0.1 p2 + 0.2 q2
Solution –
| Sl. No. | Expression | Terms | Factors |
| (a) | – 4x + 5 | -4x | -4 and x |
| 5 | 5 | ||
| (b) | – 4x + 5y | -4x | -4 and x |
| 5y | 5 and y | ||
| (c) | 5y + 3y2 | 5y | 5 and y |
| 3y2 | 3, y and y | ||
| (d) | xy + 2x2y2 | xy | x and y |
| 2x2y2 | 2, x, x, y and y | ||
| (e) | pq + q | pq | p and q |
| q | q | ||
| (f) | 1.2 ab – 2.4 b + 3.6 a | 1.2ab | 1.2, a and b |
| -2.4b | -2.4 and b | ||
| 3.6a | 3.6 and a | ||
| (g) | |||
| (h) | 0.1 p2 + 0.2 q2 | 0.1p2 | 0.1, p and p |
| 0.2q2 | 0.2, q and q |
3. Identify the numerical coefficients of terms (other than constants) in the following expressions:
(i) 5 – 3t2
(ii) 1 + t + t2 + t3
(iii) x + 2xy + 3y
(iv) 100m + 1000n
(v) – p2q2 + 7pq
(vi) 1.2 a + 0.8 b
(vii) 3.14 r2
(viii) 2 (l + b)
(ix) 0.1 y + 0.01 y2
Solution –
| Sl.No. | Expression | Terms | Coefficients |
| (i) | 5 – 3t2 | – 3t2 | -3 |
| (ii) | 1 + t + t2 + t3 | t | 1 |
| t2 | 1 | ||
| t3 | 1 | ||
| (iii) | x + 2xy + 3y | x | 1 |
| 2xy | 2 | ||
| 3y | 3 | ||
| (iv) | 100m + 1000n | 100m | 100 |
| 1000n | 1000 | ||
| (v) | – p2q2 + 7pq | -p2q2 | -1 |
| 7pq | 7 | ||
| (vi) | 1.2 a + 0.8 b | 1.2a | 1.2 |
| 0.8b | 0.8 | ||
| (vii) | 3.14 r2 | 3.142 | 3.14 |
| (viii) | 2 (l + b) | 2l | 2 |
| 2b | 2 | ||
| (ix) | 0.1 y + 0.01 y2 | 0.1y | 0.1 |
| 0.01y2 | 0.01 |
4. (a) Identify terms which contain x and give the coefficient of x.
(i) y2x + y
(ii) 13y2 – 8yx
(iii) x + y + 2
(iv) 5 + z + zx
(v) 1 + x + xy
(vi) 12xy2 + 25
(vii) 7x + xy2
Solution –
| Sl.No. | Expression | Terms | Coefficient of x |
| (i) | y2x + y | y2x | y2 |
| (ii) | 13y2 – 8yx | – 8yx | -8y |
| (iii) | x + y + 2 | x | 1 |
| (iv) | 5 + z + zx | x | 1 |
| zx | z | ||
| (v) | 1 + x + xy | xy | y |
| (vi) | 12xy2 + 25 | 12xy2 | 12y2 |
| (vii) | 7x + xy2 | 7x | 7 |
| xy2 | y2 |
(b) Identify terms which contain y2 and give the coefficient of y2.
(i) 8 – xy2
(ii) 5y2 + 7x
(iii) 2x2y – 15xy2 + 7y2
Solution –
| Sl.No. | Expression | Terms | Coefficient of y2 |
| (i) | 8 – xy2 | – xy2 | – x |
| (ii) | 5y2 + 7x | 5y2 | 5 |
| (iii) | 2x2y – 15xy2 + 7y2 | – 15xy2 | – 15x |
| 7y2 | 7 |
5. Classify into monomials, binomials and trinomials.
(i) 4y – 7x
(ii) y2
(iii) x + y – xy
(iv) 100
(v) ab – a – b
(vi) 5 – 3t
(vii) 4p2q – 4pq2
(viii) 7mn
(ix) z2 – 3z + 8
(x) a2 + b2
(xi) z2 + z
(xii) 1 + x + x2
Solution –
(i) 4y – 7x
Binomial – An expression which contains two unlike terms is called a binomial.
(ii) y2
Monomial – An expression with only one term is called a monomial.
(iii) x + y – xy
Trinomial – An expression which contains three terms is called a trinomial.
(iv) 100
Monomial – An expression with only one term is called a monomial.
(v) ab – a – b
Trinomial – An expression which contains three terms is called a trinomial.
(vi) 5 – 3t
Binomial – An expression which contains two unlike terms is called a binomial.
(vii) 4p2q – 4pq2
Binomial – An expression which contains two unlike terms is called a binomial.
(viii) 7mn
Monomial – An expression with only one term is called a monomial.
(ix) z2 – 3z + 8
Trinomial- An expression which contains three terms is called a trinomial.
(x) a2 + b2
Binomial – An expression which contains two unlike terms is called a binomial.
(xi) z2 + z
Binomial – An expression which contains two unlike terms is called a binomial.
(xii) 1 + x + x2
Trinomial – An expression which contains three terms is called a trinomial.
6. State whether a given pair of terms is of like or unlike terms.
(i) 1, 100
(ii) -7x, x
(iii) -29x, -29y
(iv) 14xy, 42yx
(v) 4m2p, 4mp2
(vi) 12xz, 12 x2y2
Solution –
(i) 1, 100
Like term – When term have the same algebraic factors, they are like terms.
(ii) -7x, x
Like term – When term have the same algebraic factors, they are like terms.
(iii) – 29x, – 29y
Unlike terms – The terms have different algebraic factors, they are unlike terms.
(iv) 14xy, 42yx
Like term – When term have the same algebraic factors, they are like terms.
(v) 4m2p, 4mp2
Unlike terms – The terms have different algebraic factors, they are unlike terms.
(vi) 12xz, 12x2z2
Unlike terms – The terms have different algebraic factors, they are unlike terms.
7. Identify like terms in the following:
(a)-xy2, -4yx2, 8x2, 2xy2, 7y2, -11x2, -100x, -11yx, 20x2y, -6x2, y, 2xy, 3x
(b) 10pq, 7p, 8q, -p2q2, -7qp, -100q, -23, 12q2p2, -5p2, 41, 2405p, 78qp, 13p2q, qp2, 701p2
Solution –
(a) -xy2, -4yx2, 8x2, 2xy2, 7y2, -11x2, -100x, -11yx, 20x2y, -6x2, y, 2xy, 3x
Like terms are:
(i) -xy2, 2xy2
(ii) -4yx2, 20x2y
(iii) 8x2, -11x2, -6x2
(iv) 7y, y
(v) -100x, 3x
(vi) -11yx, 2xy
(b) 10pq, 7p, 8q, -p2q2, -7qp, -100q, -23, 12q2p2, -5p2, 41, 2405p, 78qp, 13p2q, qp2, 701p2
Like terms are:
(i) 10pq, – 7qp, 78qp
(ii) 7p, 2405p
(iii) 8q, -100q
(iv) -p2q2, 12 q2p2
(v) -23, 41
(vi) -5p2, 701p2
(vii) 13p2q, qp2

