NCERT Solutions Class 8 Mathematics
Chapter – 9 (Algebraic Expressions and Identities)
The NCERT Solutions in English Language for Class 8 Mathematics Chapter – 9 Algebraic Expressions and Identities Exercise 9.3 has been provided here to help the students in solving the questions from this exercise.
Chapter 9: Algebraic Expressions and Identities
- NCERT Solution Class 8 Maths Ex – 9.1
- NCERT Solution Class 8 Maths Ex – 9.2
- NCERT Solution Class 8 Maths Ex – 9.4
- NCERT Solution Class 8 Maths Ex – 9.5
Exercise – 9.3
1. Carry out the multiplication of the expressions in each of the following pairs.
(i) 4p, q + r
(ii) ab, a – b
(iii) a + b, 7a²b²
(iv) a2 – 9, 4a
(v) pq + qr + rp, 0
Solution –
(i) 4p, q + r
= 4p(q + r)
= 4pq + 4pr
(ii) ab, a – b
= ab(a – b)
= a2b – ab2
(iii) a + b, 7a²b²
= (a + b) (7a2b2)
= 7a3b2 + 7a2b3
(iv) a2 – 9, 4a
= (a2 – 9)(4a)
= 4a3 – 36a
(v) pq + qr + rp, 0
= (pq + qr + rp) × 0
= 0 ( Anything multiplied by zero is zero )
2. Complete the table.
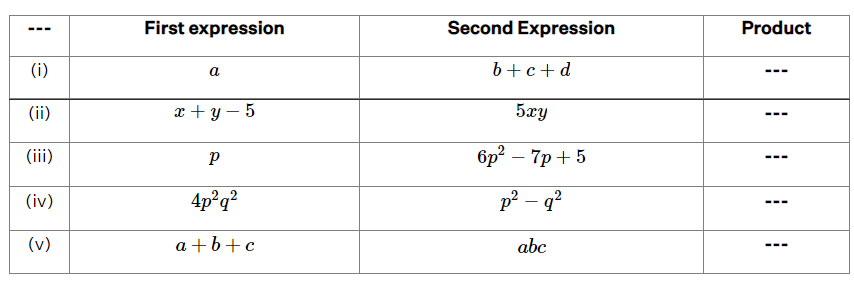
Solution –
| First expression | Second Expression | Product | |
| (i) | a | b + c + d | ab + ac + ad |
| (ii) | x + y – 5 | 5xy | 5x2y + 5xy2 – 25xy |
| (iii) | p | 6 p2 – 7 p + 5 | 6 p3 – 7 p2 + 5 p |
| (iv) | 4 p2q2 | p2 – q2 | 4 p4q2 – 4 p2q4 |
| (v) | a + b + c | abc | a2bc + ab2c + abc2 |
3. Find the product.
(i) a2 × (2a22) × (4a26)
(ii) ×
(iii) ×
(iv) (x) × (x2) × (x3) × (x4)
Solution –
(i) a2 × (2a22) × (4a26)
= (2 × 4) ( a2 × a22 × a26 )
= 8 × a2 + 22 + 26
= 8a50
(ii) ×
= ( x × x2 × y × y2 )
= x3y3
(iii) ×
= (p × p3× q3 × q)
= – 4p4q4
(iv) (x) × (x2) × (x3) × (x4)
= x 1 + 2 + 3 + 4
= x10
4.
(a) Simplify 3x (4x – 5) + 3 and find its values for
(i) x = 3
(ii) x =
(b) Simplify a (a2+ a + 1) + 5 and find its value for
(i) a = 0,
(ii) a = 1
(iii) a = – 1
Solution –
(a) Simplify 3x (4x – 5) + 3 and find its values for
= 3x (4x – 5) + 3
= 3x (4x) – 3x ( 5) + 3
= 12x2 – 15x + 3
(i) For x = 3,
= 12x2 -15x + 3
= 12(3)2 -15(3) + 3
= 108 – 45 + 3
= 66
(ii) For x =
= 12x2 -15x + 3
= 12 – 15
+ 3
= 3 – + 3
=
=
(b) a(a2 + a + 1) + 5
=a3 + a2 + a + 5
(i) For a = 0,
a3 + a2 + a + 5
= 0 + 0 + 0 + 5
= 5
(ii) For a = 1,
a3 + a2 + a + 5
= (1)3 + (1)2 + 1 + 5
= 1 + 1 + 1 + 5
= 8
(iii) For a = -1,
a3 + a2 + a + 5
= (-1)3 + (-1)2 + (-1) + 5
= – 1 + 1 -1 + 5
= 4
5.
(a) Add: p ( p – q), q ( q – r) and r ( r – p)
(b) Add: 2x (z – x – y) and 2y (z – y – x)
(c) Subtract: 3l (l – 4 m + 5 n) from 4l ( 10 n – 3 m + 2 l )
(d) Subtract: 3a (a + b + c ) – 2 b (a – b + c) from 4c ( – a + b + c )
Solution –
(a) Add: p ( p – q), q ( q – r) and r ( r – p)
= p ( p – q) + q ( q – r) + r ( r – p)
= (p2 – pq) + (q2 – qr) + (r2 – pr)
= p2 + q2 + r2 – pq – qr – pr
(b) Add: 2x (z – x – y) and 2y (z – y – x)
= 2x (z – x – y) + 2y (z – y – x)
= (2xz – 2x2 – 2xy) + (2yz – 2y2 – 2xy)
= 2xz – 4xy + 2yz – 2x2 – 2y2
(c) Subtract: 3l (l – 4 m + 5 n) from 4l ( 10 n – 3 m + 2 l )
= 4l ( 10 n – 3 m + 2 l ) – 3l (l – 4 m + 5 n)
= (40ln – 12lm + 8l2) – (3l2 – 12lm + 15ln)
= 40ln – 12lm + 8l2 – 3l2 +12lm -15 ln
= 25 ln + 5l2
(d) Subtract: 3a (a + b + c ) – 2 b (a – b + c) from 4c ( – a + b + c )
= 4c ( – a + b + c ) – (3a (a + b + c ) – 2 b (a – b + c))
= (-4ac + 4bc + 4c2) – (3a2 + 3ab + 3ac – ( 2ab – 2b2 + 2bc ))
=-4ac + 4bc + 4c2 – (3a2 + 3ab + 3ac – 2ab + 2b2 – 2bc)
= -4ac + 4bc + 4c2 – 3a2 – 3ab – 3ac +2ab – 2b2 + 2bc
= -7ac + 6bc + 4c2 – 3a2 – ab – 2b2

