NCERT Solutions Class 8 Mathematics
Chapter – 3 (Understanding Quadrilaterals)
The NCERT Solutions in English Language for Class 8 Mathematics Chapter – 3 Understanding Quadrilaterals Exercise 3.3 has been provided here to help the students in solving the questions from this exercise.
Chapter 3: Understanding Quadrilaterals
- NCERT Solution Class 8 Maths Ex – 3.1
- NCERT Solution Class 8 Maths Ex – 3.2
- NCERT Solution Class 8 Maths Ex – 3.4
Exercise – 3.3
1. Given a parallelogram ABCD. Complete each statement along with the definition or property used.
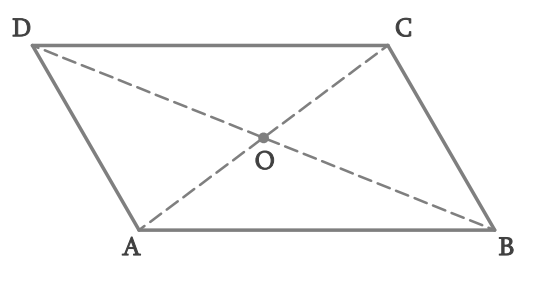
(i) AD = ______
(ii) ∠DCB = ______
(iii) OC = ______
(iv) m ∠DAB + m ∠CDA = ______
Solution –
(i) AD = BC (Opposite sides of a parallelogram are equal)
(ii) ∠DCB = ∠DAB (Opposite angles of a parallelogram are equal)
(iii) OC = OA (Diagonals of a parallelogram are equal)
(iv) m ∠DAB + m ∠CDA = 180°
2. Consider the following parallelograms. Find the values of the unknown x, y, z

Solution –
(i) y = 100° (opposite angles of a parallelogram)
x + 100° = 180° (adjacent angles of a parallelogram)
⇒ x = 180° – 100° = 80°
x = z = 80° (opposite angles of a parallelogram)
∴ x = 80°, y = 100° and z = 80°
(ii) 50° + x = 180°
⇒ x = 180° – 50° = 130° (adjacent angles of a parallelogram)
x = y = 130° (opposite angles of a parallelogram)
x = z = 130° (corresponding angle)
(iii) x = 90° (vertical opposite angles)
x + y + 30° = 180° (angle sum property of a triangle)
⇒ 90° + y + 30° = 180°
⇒ y = 180° – 120° = 60°
also, y = z = 60° (alternate angles)
(iv) z = 80° (corresponding angle)
z = y = 80° (alternate angles)
x + y = 180° (adjacent angles)
⇒ x + 80° = 180°
⇒ x = 180° – 80° = 100°
(v) y = 112° {opposite angles of a parallelogram}
x = 180° – (y + 40°) {angle sum property of a triangle}
x = 180° – (112°+ 40°) = 180° – 162° = 28°
z = 28° {alternate angles}
3. Can a quadrilateral ABCD be a parallelogram if
(i) ∠D + ∠B = 180°?
(ii) AB = DC = 8 cm, AD = 4 cm and BC = 4.4 cm?
(iii) ∠A = 70° and ∠C = 65°?
Solution –
(i) Yes,
The quadrilateral ABCD be a parallelogram if ∠D + ∠B = 180°, it should also fulfilled some conditions which are:
(a) The sum of the adjacent angles should be 180°.
(b) Opposite angles must be equal.
(ii) No, opposite sides should be of the same length. Here, AD ≠ BC
(iii) No, opposite angles should be of the same measures. ∠A ≠ ∠C
4. Draw a rough figure of a quadrilateral that is not a parallelogram but has exactly two opposite angles of equal measure.
Solution –
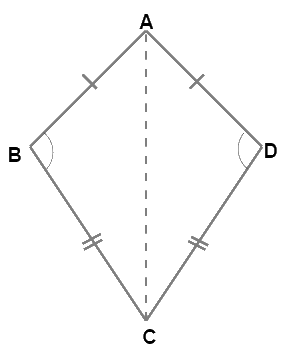
ABCD is a figure of quadrilateral that is not a parallelogram but has exactly two opposite angles, that is, ∠B = ∠D of equal measure. It is not a parallelogram because ∠A ≠ ∠C.
5. The measures of two adjacent angles of a parallelogram are in the ratio 3 : 2. Find the measure of each of the angles of the parallelogram.
Solution –
Let the measures of two adjacent angles ∠A and ∠B be 3x and 2x, respectively in parallelogram ABCD.
∠A + ∠B = 180°
⇒ 3x + 2x = 180°
⇒ 5x = 180°
⇒ x = 36°
We know that opposite sides of a parallelogram are equal.
∠A = ∠C = 3x = 3 × 36° = 108°
∠B = ∠D = 2x = 2 × 36° = 72°
6. Two adjacent angles of a parallelogram have equal measure. Find the measure of each of the angles of the parallelogram.
Solution –
Let ABCD be a parallelogram.
Sum of adjacent angles of a parallelogram = 180°
∠A + ∠B = 180°
⇒ 2∠A = 180°
⇒ ∠A = 90°
also, 90° + ∠B = 180°
⇒ ∠B = 180° – 90° = 90°
∠A = ∠C = 90°
∠B = ∠D = 90°
7. The adjacent figure HOPE is a parallelogram. Find the angle measures x, y and z. State the properties you use to find them.
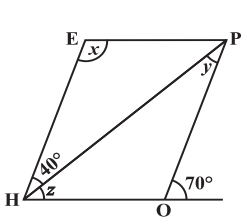
Solution –
y = 40° (alternate interior angle)
∠P = 70° (alternate interior angle)
∠P = ∠H = 70° (opposite angles of a parallelogram)
z = ∠H – 40°= 70° – 40° = 30°
∠H + x = 180°
⇒ 70° + x = 180°
⇒ x = 180° – 70° = 110°
8. The following figures GUNS and RUNS are parallelograms. Find x and y. (Lengths are in cm)
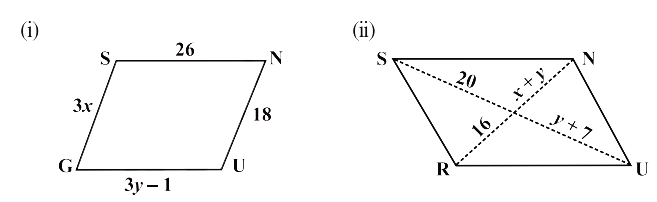
Solution –
(i) SG = NU and SN = GU (opposite sides of a parallelogram are equal)
3x = 18
⇒ x =6
3y – 1 = 26
⇒ 3y = 26 + 1
⇒ y = 9
x = 6 and y = 9
(ii) 20 = y + 7 and 16 = x + y (diagonals of a parallelogram bisect each other)
y + 7 = 20
⇒ y = 20 – 7 = 13 and,
x + y = 16
⇒ x + 13 = 16
⇒ x = 16 – 13 = 3
x = 3 and y = 13
9. In the above figure both RISK and CLUE are parallelograms. Find the value of x.
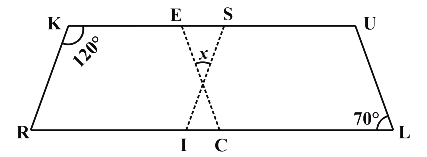
Solution –
∠K + ∠R = 180° (adjacent angles of a parallelogram are supplementary)
⇒ 120° + ∠R = 180°
⇒ ∠R = 180° – 120° = 60°
also, ∠R = ∠SIL (corresponding angles)
⇒ ∠SIL = 60°
also, ∠ECR = ∠L = 70° (corresponding angles)
x + 60° + 70° = 180° (angle sum of a triangle)
⇒ x + 130° = 180°
⇒ x = 180° – 130° = 50°
10. Explain how this figure is a trapezium. Which of its two sides are parallel? (Fig 3.32)
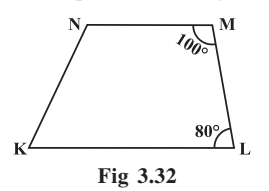
Solution –
In the given figure KLMN
Since two pair of adjacent angles which form pairs of consecutive interior angles are supplementary,
i.e., ∠L + ∠M = 180°
Thus, 80° + 100° = 180°
Therefore, NM is parallel to KL
Hence, KLMN is a trapezium as it has a pair of parallel sides KL and NM.
11. Find m∠C in Fig 3.33 if AB || DC.
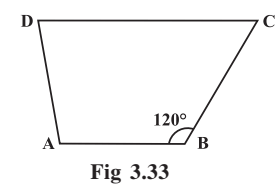
Solution –
Given figure ABCD is a Trapezium, in which AB is parallel to DC .
Here,
∠B + ∠C = 180° (pair of adjacent angles are supplementary)
120° + ∠C = 180°
∠C = 180° – 120°
∠C = 60°
Therefore, m∠C = 60°
12. Find the measure of ∠P and ∠S if SP || RQ ? in Fig 3.34. (If you find m∠R, is there more than one method to find m∠P?)
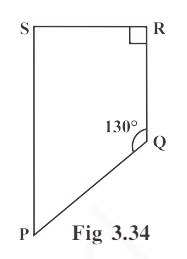
Solution – Given SP is parallel to RQ and SR is the traversal drawn to these lines. Hence, SPQR is a trapezium.
∠S + ∠R = 180°
∠S + 90° = 180° [Since, ∠R = 90° in the given figure]
∠S = 180° – 90°
∠S = 90°
Using the angle sum property of a quadrilateral,
∠S + ∠P + ∠Q + ∠R = 360°
90° + ∠P + 130° + 90° = 360°
∠P + 310° = 360°
∠P = 360° – 310°
∠P = 50°

