NCERT Solutions Class 8 Mathematics
Chapter – 3 (Understanding Quadrilaterals)
The NCERT Solutions in English Language for Class 8 Mathematics Chapter – 3 Understanding Quadrilaterals Exercise 3.1 has been provided here to help the students in solving the questions from this exercise.
Chapter 3: Understanding Quadrilaterals
- NCERT Solution Class 8 Maths Ex – 3.2
- NCERT Solution Class 8 Maths Ex – 3.3
- NCERT Solution Class 8 Maths Ex – 3.4
Exercise – 3.1
1. Given here are some figures.
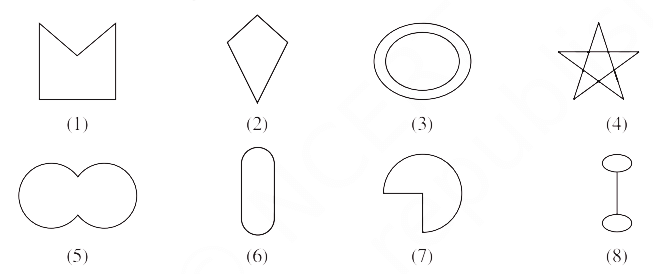
Classify each of them on the basis of the following.
(a) Simple curve
(b) Simple closed curve
(c) Polygon
(d) Convex polygon
(e) Concave polygon
Solution –
(a) Simple curve – 1, 2, 5, 6 and 7
(b) Simple closed curve – 1, 2, 5, 6 and 7
(c) Polygon – 1 and 2
(d) Convex polygon – 2
(e) Concave polygon – 1
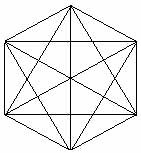
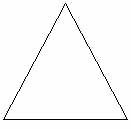
2. How many diagonals does each of the following have?
(a) A convex quadrilateral
(b) A regular hexagon
(c) A triangle
Solution –
(a) A convex quadrilateral – 2

(b) A regular hexagon – 9
(c) A triangle – 0
3. What is the sum of the measures of the angles of a convex quadrilateral? Will this property hold if the quadrilateral is not convex? (Make a non-convex quadrilateral and try!)
Solution –
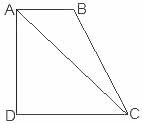
Let ABCD be a convex quadrilateral.
From the figure, we infer that the quadrilateral ABCD is formed by two triangles,
i.e. ΔADC and ΔABC.
Since we know that sum of the interior angles of a triangle is 180°,
the sum of the measures of the angles is 180° + 180° = 360°

Let us take another quadrilateral ABCD which is not convex .
Join BC, such that it divides ABCD into two triangles ΔABC and ΔBCD. In ΔABC,
∠1 + ∠2 + ∠3 = 180° (angle sum property of triangle)
In ΔBCD,
∠4 + ∠5 + ∠6 = 180° (angle sum property of triangle)
∴ ∠1 + ∠2 + ∠3 + ∠4 + ∠5 + ∠6 = 180° + 180°
⇒ ∠1 + ∠2 + ∠3 + ∠4 + ∠5 + ∠6 = 360°
⇒ ∠A + ∠B + ∠C + ∠D = 360°
Thus, this property holds if the quadrilateral is not convex.
4. Examine the table. (Each figure is divided into triangles and the sum of the angles deduced from that.)
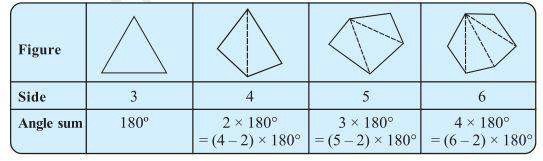
What can you say about the angle sum of a convex polygon with number of sides?
(a) 7
(b) 8
(c) 10
(d) n
Solution –
The angle sum of a polygon having side n = (n-2)×180°
(a) 7
Here, n = 7
Thus, angle sum = (7 – 2)×180°
= 5 × 180°
= 900°
(b) 8
Here, n = 8
Thus, angle sum = (8 – 2) × 180°
= 6 × 180°
= 1080°
(c) 10
Here, n = 10
Thus, angle sum = (10 – 2) × 180°
= 8 × 180°
= 1440°
(d) n
Here, n = n
Thus, angle sum = (n – 2) × 180°
5. What is a regular polygon?
State the name of a regular polygon of
(i) 3 sides
(ii) 4 sides
(iii) 6 sides
Solution –
Regular polygon: A polygon having sides of equal length and angles of equal measures is called a regular polygon. A regular polygon is both equilateral and equiangular.
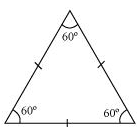
(i) A regular polygon of 3 sides is called an equilateral triangle.
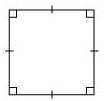
(ii) A regular polygon of 4 sides is called a square.
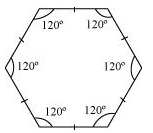
(iii) A regular polygon of 6 sides is called a regular hexagon.
6. Find the angle measure of x in the following figures.
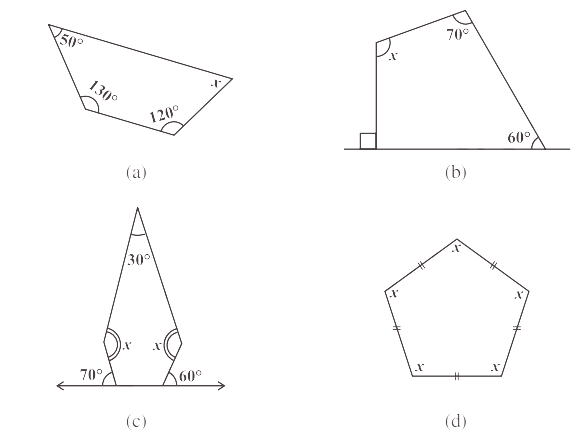
Solution –
(a) The figure has 4 sides.
Hence, it is a quadrilateral.
Sum of angles of the quadrilateral = 360°
⇒ 50° + 130° + 120° + x = 360°
⇒ 300° + x = 360°
⇒ x = 360° – 300° = 60°
(b) The figure has 4 sides.
Hence, it is a quadrilateral.
Also, one side is perpendicular forming a right angle.
Sum of angles of the quadrilateral = 360°
⇒ 90° + 70° + 60° + x = 360°
⇒ 220° + x = 360°
⇒ x = 360° – 220° = 140°
(c) The figure has 5 sides.
Hence, it is a pentagon.
Sum of angles of the pentagon = 540°
Two angles at the bottom are a linear pair.
∴ 180° – 70° = 110°
180° – 60° = 120°
⇒ 30° + 110° + 120° + x + x = 540°
⇒ 260° + 2x = 540°
⇒ 2x = 540° – 260° = 280°
⇒ 2x = 280°
= 140°
(d) The figure has 5 equal sides.
Hence, it is a regular pentagon.
Thus, all its angles are equal.
5x = 540°
⇒ x = 108°
7.
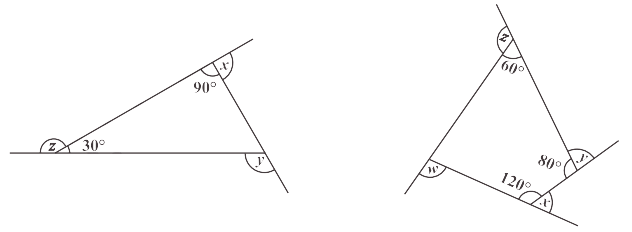
(a) Find x + y + z
(b) Find x + y + z + w
Solution –
(a) Sum of all angles of triangle = 180°
One side of triangle = 180°- (90° + 30°) = 60°
x + 90° = 180° ⇒ x = 180° – 90° = 90°
y + 60° = 180° ⇒ y = 180° – 60° = 120°
z + 30° = 180° ⇒ z = 180° – 30° = 150°
x + y + z = 90° + 120° + 150° = 360°
(b) Sum of all angles of quadrilateral = 360°
One side of quadrilateral = 360°- (60° + 80° + 120°) = 360° – 260° = 100°
x + 120° = 180° ⇒ x = 180° – 120° = 60°
y + 80° = 180° ⇒ y = 180° – 80° = 100°
z + 60° = 180° ⇒ z = 180° – 60° = 120°
w + 100° = 180° ⇒ w = 180° – 100° = 80°
x + y + z + w = 60° + 100° + 120° + 80° = 360°

