NCERT Solutions Class 10 Maths
Chapter – 6 (Triangles)
The NCERT Solutions in English Language for Class 10 Mathematics Chapter – 6 Triangles Exercise 6.6 has been provided here to help the students in solving the questions from this exercise.
Chapter : 6 Triangles
- NCERT Class 10 Maths Solution Ex – 6.1
- NCERT Class 10 Maths Solution Ex – 6.2
- NCERT Class 10 Maths Solution Ex – 6.3
- NCERT Class 10 Maths Solution Ex – 6.4
- NCERT Class 10 Maths Solution Ex – 6.5
Exercise – 6.6
1. In Figure, PS is the bisector of ∠ QPR of ∆ PQR. Prove that QS/PQ = SR/PR
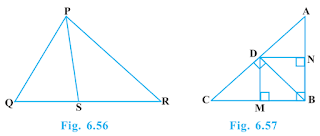
Solution –
Given : PS is the bisector of ∠QPR of ∆PQR.
Now, Draw RT SP || to meet QP produced in T.
Proof :
∵ RT SP || and transversal PR intersects them
∴ ∠1 = ∠2 (Alternate interior angle) ————— (i)
∴ RT SP || and transversalQT intersects them
∴ ∠3 = ∠4 (Corresponding angle) ————— (ii)
But ∠1 = ∠3 (Given)
∴ ∠2 = ∠4 [From Eqs. (i) and (ii)]
∴ PT = PR …(iii) (∵ Sides opposite to equal angles of a triangle are equal)
Now, in ∆QRT,
PS || RT (By construction)
∴ QS/SR = PQ/PT (By basic proportionally theorem)
⇒ QS/SR = PQ/PR [From Eq. (iii)]
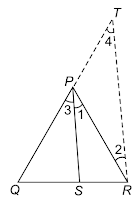
2. In Fig. 6.57, D is a point on hypotenuse AC of ∆ABC, such that BD ⊥AC, DM ⊥ BC and DN ⊥ AB. Prove that:
(i) DM2 = DN . MC
(ii) DN2 = DM . AN.
Solution –

Given : D is a point on hypotenuse AC of ∆ABC, DM ⊥ BC and DN ⊥ AB.
Now, join NM.
Let BD and NM intersect at O.
(i) In ∆DMC and ∆NDM,
∠DMC = ∠NDM (Each equal to 90°)
∠MCD = ∠DMN
Let MCD = ∠1
Then,
∠MDC = 90° − (90°-∠1)
= ∠1 (∵∠MCD + ∠MDC + ∠DMC = 180°)
∴ ∠ODM = 90° − (90° − ∠1)
= ∠1
⇒ ∠DMN = ∠1
∴ ∆DMO ~ ∆NDM (AA similarity criterion)
∴ DM/ND = MC/DM (Corresponding sides of the similar triangles are proportional)
⇒ DM2 = MC ND
(ii) In ∆DNM and ∆NAD,
∠NDM = ∠AND (Each equal to 90°)
∠DNM = ∠NAD
Let ∠NAD = ∠2
Then,
∠NDA = 90° − ∠2
∵∠NDA + ∠DAN + ∠DNA = 180°
∴ ∠ODN = 90° − (90° − ∠2) = ∠2
∴ ∠DNO = ∠2
∴ ∆DNM ~ ∆NAD (AA similarity criterion)
∴ DN/NA = DM/ND
⇒ DN/AN = DM/DN
⇒ DN2 = DM×AN
3. In Figure, ABC is a triangle in which ∠ABC > 90° and AD ⊥ CB produced. Prove that
AC2= AB2+ BC2+ 2 BC.BD.

Solution –
Given : ABC is a triangle in which ∠ABC> 90° and AD ⊥ CB produced.
Proof : In right ADC,
∠D = 90°
AC2 = AD2 + DC2 (By Pythagoras theorem)
= AD2 + (BD + BC)2 [∵DC = DB + BC]
= (AD2 + DB2) + BC2 + 2BD.BC [∵ (a + b)2 = a2 + b2 + 2ab]
= AB2 + BC2 + 2BC.BD [∵In right ADB with ∠D = 90°, AB2 = AD2 + DB2] (By Pythagoras theorem)
4. In Figure, ABC is a triangle in which ∠ ABC < 90° and AD ⊥ BC. Prove that AC2= AB2+ BC2 – 2 BC.BD.

Solution –
Given : ABC is a triangle in which ∠ABC < 90° and AD ⊥ BC.
Proof : In right △ADC, ∠D = 90°
AC2 = AD2 + DC2 (By Pythagoras theorem)
= AD2 + (BC – BD)2 [∵ BC = BD + DC]
= AD2 + (BC – BD)2 (BC = BD + DC)
= AD2 + BC2 + BD2 – 2BC.BD [∵ (a + b)2 = a2 + b2 + 2ab]
= (AD2 + BD2) + BC2 – 2BC . BD
= AB2 + BC2 – 2BC . BD {In right △ADB with ∠D = 90°, AB2 = AD2 + BD2} (By Pythagoras theorem)
5. In Figure, AD is a median of a triangle ABC and AM ⊥ BC. Prove that :
(i) AC2 = AD2 + BC.DM + 2 (BC/2) 2
(ii) AB2 = AD2 – BC.DM + 2 (BC/2) 2
(iii) AC2 + AB2 = 2 AD2 + ½ BC2

Solution –
Given : AD is a median of a ∆ABC and AM ⊥ BC.
Proof :
(i) In right ∆AMC, ∠M = 90°
AC2 = AM2 + MC2 (By Pythagoras theorem)
AC2 = AM2 + (MD + DC) 2 (∵ MC = MD + DC)
AC2 = AM2 + MD2 + DC2 + 2MD.DC (∵ (a + b)2 = a2 + b2 + 2ab)
AC2 = AD2 + DC2 + 2MD.DC
[∵ In right △AMD with ∠M = 90°, AD2 = AM2 + MD2} (By Pythagoras theorem]
Since, DC = BC/2,
thus, we get,
AC2 = AD2 + (BC/2) 2 + 2MD.(BC/2) 2
AC2 = AD2 + (BC/2) 2 + 2MD × BC
Hence, proved.
(ii) In right ∆AMB, ∠M = 90°
AB2 = AM2 + MB2
= (AD2 − DM2) + MB2 (By Pythagoras theorem)
= (AD2 − DM2) + (BD − MD) 2 (∵ BD = BM + MD)
= AD2 − DM2 + BD2 + MD2 − 2BD × MD (∵ (a – b)2 = a2 + b2 – 2ab)
= AD2 + BD2 − 2BD × MD
Since, 2BD = BC, AD is a median of ∆ABC
thus, we get,
= AD2 + (BC/2)2 – 2(BC/2) MD
= AD2 + (BC/2)2 – BC MD
Hence, proved.
(iii) By applying Pythagoras Theorem in ∆ABM, we get,
AM2 + MB2 = AB2 ——————– (i)
By applying Pythagoras Theorem in ∆AMC, we get,
AM2 + MC2 = AC2 ——————– (ii)
Adding both the equations (i) and (ii), we get,
2AM2 + MB2 + MC2 = AB2 + AC2
2AM2 + (BD − DM) 2 + (MD + DC) 2 = AB2 + AC2
2AM2+BD2 + DM2 − 2BD.DM + MD2 + DC2 + 2MD.DC = AB2 + AC2
2AM2 + 2MD2 + BD2 + DC2 + 2MD (− BD + DC) = AB2 + AC2
2(AM2+ MD2) + (BC/2) 2 + (BC/2) 2 + 2MD (-BC/2 + BC/2) 2 = AB2 + AC2
2AD2 + BC2/2 = AB2 + AC2
6. Prove that the sum of the squares of the diagonals of parallelogram is equal to the sum of the squares of its sides.
Solution –
Given : ABCD is a parallelogram whose diagonals are AC and BD.
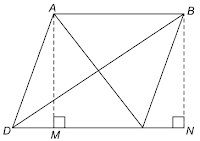
Now, draw AM⊥DC and BN⊥D (produced).
Proof : In right ∆AMD and ∆BNC,
AD = BC (Opposite sides of a parallelogram)
AM = BN (Both are altitudes of the same parallelogram to the same base) ,
△AMD ⩭ △BNC (RHS congruence criterion)
MD = NC (CPCT) ————– (i)
In right △BND, ∠N = 90°
BD2 = BN2 + DN2(By Pythagoras theorem)
= BN2 + (DC + CN)2 (∵ DN = DC + CN)
= BN2 + DC2 + CN2 + 2DC.CN [∵ (a + b)2 = a2 + b2 + 2ab]
= (BN2 + CN2) + DC2 + 2DC.CN
= BC2 + DC2 + 2DC.CN ————– (ii) (∵In right △BNC with ∠N = 90°)
BN2 + CN2 = BC2 (By Pythagoras theorem)
In right △AMC, ∠M = 90°
AC2 = AM2 + MC2(∵MC = DC – DM)
= AM2 + (DC – DM)2 [∵ (a + b)2 = a2 + b2 + 2ab]
= AM2 + DC2 + DM2 – 2DC.DM
= (AM2 + DM2) + DC2 – 2DC.DM
= AD2 + DC2 – 2DC.DM [∵ In right triangle AMD with ∠M = 90°, AD2 = AM2 + DM2 (By Pythagoras theorem)]
= AD2 + AB2 = 2DC.CN ————– (iii) [∵ DC = AB, opposite sides of parallelogram and BM = CN from eq (i)]
Now, on adding Eqs. (iii) and (ii), we get
AC2 + BD2 = (AD2 + AB2) + (BC2 + DC2)
= AB2 + BC2 + CD2 + DA2
7. In Figure, two chords AB and CD intersect each other at the point P. Prove that :
(i) ∆APC ~ ∆ DPB
(ii) AP . PB = CP . DP
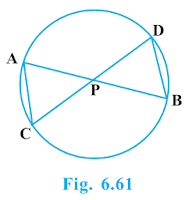
Solution –
Given : two chords AB and CD intersects each other at the point P.
Proof:
(i) ∆APC and ∆DPB
∠APC = ∠DPB (Vertically opposite angles)
∠CAP = ∠BDP (Angles in the same segment for chord CB)
Therefore,
∆APC ∼ ∆DPB (AA similarity criterion)
(ii) ∆APC ~ ∆DPB
In the above, we have proved that ∆APC ∼ ∆DPB
We know that the corresponding sides of similar triangles are proportional.
∴ AP/DP = PC/PB = CA/BD
⇒ AP/DP = PC/PB
∴ AP. PB = PC. DP
8. In Fig. 6.62, two chords AB and CD of a circle intersect each other at the point P (when produced) outside the circle. Prove that:
(i) ∆ PAC ~ ∆ PDB
(ii) PA . PB = PC . PD.
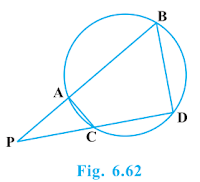
Solution –
Given : two chords AB and CD of a circle intersect each other at the point P (when produced) out the circle.
Proof :
(i) In ∆PAC and ∆PDB,
∠P = ∠P (Common Angles)
As we know, exterior angle of a cyclic quadrilateral is ∠PCA and ∠PBD is opposite interior angle, which are both equal.
∠PAC = ∠PDB
Thus, ∆PAC ∼ ∆PDB(AA similarity criterion)
(ii) ∆PAC ~ ∆PDB
We have already proved above,
∆APC ∼ ∆DPB
We know that the corresponding sides of similar triangles are proportional.
Therefore,
AP/DP = PC/PB = CA/BD
AP/DP = PC/PB
∴ AP. PB = PC. DP
9. In Figure, D is a point on side BC of ∆ ABC such that BD/CD = AB/AC. Prove that AD is the bisector of ∠ BAC.
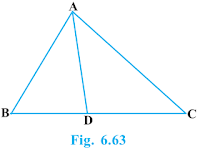
Solution –
Given : D is a point on side BC of ∆ABC such that BD/CD = AB/AC
Now, from BA produce cut off AE = A. JoinCE.
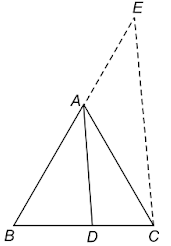
By using the converse of basic proportionality theorem, we get,
AD || PC
∠BAD = ∠APC (Corresponding angles) ————— (i)
And,
∠DAC = ∠ACP (Alternate interior angles) ————— (ii)
By the new figure, we have;
AP = AC
⇒ ∠APC = ∠ACP ————— (iii)
On comparing equations (i), (ii), and (iii), we get,
∠BAD = ∠APC
Therefore, AD is the bisector of the angle BAC.
Hence, proved.
10. Nazima is fly fishing in a stream. The tip of her fishing rod is 1.8 m above the surface of the water and the fly at the end of the string rests on the water 3.6 m away and 2.4 m from a point directly under the tip of the rod. Assuming that her string (from the tip of her rod to the fly) is taut, how much string does she have out (see Figure)? If she pulls in the string at the rate of 5 cm per second, what will be the horizontal distance of the fly from her after 12 seconds?
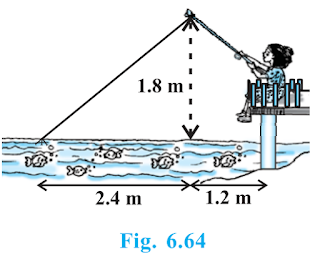
Solution – Length of the string that she has out
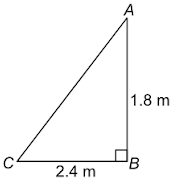
To find AC, we have to use Pythagoras theorem in ∆ABC, is such way;
AC2 = AB2+ BC2
AC2 = (1.8 m) 2 + (2.4 m) 2
AC2 = (3.24 + 5.76) m2
AC2 = 9.00 m2
⟹ AC = √9 m = 3m
Thus, the length of the string out is 3 m.
Length of string out after 12 seconds is AD.
AD = AC − String pulled by Nazima in 12 seconds
= (3.00 −12 × 5 cm)
= (3.00 − 60 cm)
= (3.00 − 0.6) m
= 2.4 m
In ∆ADB, by Pythagoras Theorem,
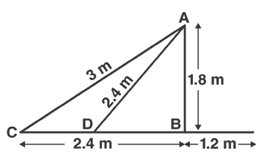
AD2 = AB2 + BD2
(2.4 m) 2 = (1.8 m) 2 + BD2
BD2 = (5.76 − 3.24) m2 = 2.52 m2
BD = 1.587 m
Horizontal distance of fly from Nazima after 12 sec = BD + 1.2 m
= (1.587 + 1.2) m = 2.787 m
= 2.79 m (Approximate)

