NCERT Solutions Class 10 Maths Chapter 13 Surface Areas and Volumes Ex 13.4

NCERT Solutions Class 10 Maths 
Chapter – 13 (Surface Areas and Volumes) 

The NCERT Solutions in English Language for Class 10 Mathematics Chapter – 13 Surface Areas and Volumes Exercise 13.4 has been provided here to help the students in solving the questions from this exercise. 

Chapter : 13 Surface Areas and Volumes

• NCERT Class 10 Maths Solution Ex – 13.1
• NCERT Class 10 Maths Solution Ex – 13.2
• NCERT Class 10 Maths Solution Ex – 13.3
• NCERT Class 10 Maths Solution Ex – 13.5

Exercise – 13.4

1. A drinking glass is in the shape of a frustum of a cone of height 14 cm. The diameters of its two circular ends are 4 cm and 2 cm. Find the capacity of the glass.
Solution –

Radius (r₁) of the upper base = 4/2 = 2 cm
Radius (r₂) of lower the base = 2/2 = 1 cm
Height = 14 cm
Now,
The capacity of glass = Volume of the frustum of the cone
Capacity of glass = (⅓) × π × h (r₁² + r₂² + r₁r₂)
= (⅓) × (22/7) × (14) (2² + 1² +  (2)(1))
= (44/3) × (4 + 1 + 2)
= 308/3
=
∴ The capacity of the glass = 102×(⅔) cm³

2. The slant height of a frustum of a cone is 4 cm, and the perimeters (circumference) of its circular ends are 18 cm and 6 cm. Find the surface area of the frustum.
Solution –

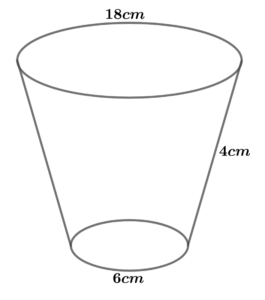
Slant height (l) = 4 cm
Circumference of upper circular end of the frustum = 18 cm
∴ 2πr₁ = 18
r₁ = 9/π
Similarly, the circumference of the lower end of the frustum = 6 cm
∴ 2πr₂ = 6
r₂ = 3/π
Now, the surface area of the frustum = π(r₁ + r₂) × l
= π(9/π + 3/π) × 4
= 12 × 4
= 48 cm²
The curved surface area of the frustum is 48 cm².

3. A fez, the cap used by the Turks, is shaped like the frustum of a cone (see Fig.). If its radius on the open side is 10 cm, the radius at the upper base is 4 cm, and its slant height is 15 cm, find the area of material used for making it.

Solution –  Given,

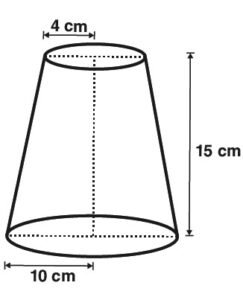
For the lower circular end, radius (r₁) = 10 cm
For the upper circular end, radius (r₂) = 4 cm
Slant height (l) of frustum = 15 cm
Now,
The area of material to be used for making the fez = CSA of frustum + Area of the upper circular end
CSA of frustum = π(r₁ + r₂)×l
= π(10 + 4)×15
= 210π
And, the Area of the upper circular end = πr₂²
= π4²
= 16π
The area of material to be used for making the fez = 210π + 16π
= (226 × 22)/7

= 710.29 cm²
710.29 cm² of the material used for making Fez.

4. A container, opened from the top and made up of a metal sheet, is in the form of a frustum of a cone of height 16 cm with radii of its lower and upper ends as 8 cm and 20 cm, respectively. Find the cost of the milk which can completely fill the container at the rate of Rs. 20 per litre. Also, find the cost of the metal sheet used to make the container if it costs Rs. 8 per 100 cm².
Solution – Given,

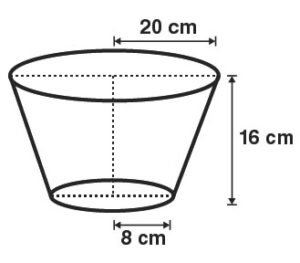
r₁ = 20 cm,
r₂ = 8 cm and
h = 16 cm
∴ Volume of the frustum = (⅓)×π×h(r₁²+r₂²+r₁r₂)
= 1/3 ×3.14 ×16((20)²+(8)²+(20)(8))
= 1/3 ×3.14 ×16(400 + 64 + 160)
= 10449.92 cm³
= 10.45 lit
It is given that the rate of milk = Rs. 20/litre
So, the cost of milk = 20×volume of the frustum
= 20×10.45
= Rs. 209
Now, the slant height l = √[(r₁ – r₂)² + h²]
l = √[(20 – 8)² + (16)²]
l = √[144 + 256]
l = √400
l = 20 cm
Area of metal sheet required to make the container = CSA of frustum of the cone + Area of lower circular end
= π (r₁ + r₂) l + πr₂²
= π [ (r₁ + r₂) l + r₂²]
= 3.14 × [ (20 + 8) × 20 + (8)²]
= 3.14 × [560 + 64 ]
= 3.14 × 624 cm²
= 1959.36 cm²
Cost of 100 cm² of metal sheet = ₹ 8
Therefore, cost of 1959.36 cm² of metal sheet = ₹ (8/100) × 1959.36
= ₹ 156.7488
= ₹ 156.75

5. A metallic right circular cone 20 cm high and whose vertical angle is 60° is cut into two parts at the middle of its height by a plane parallel to its base. If the frustum so obtained is drawn into a wire of diameter 1/16 cm, find the length of the wire.
Solution –  The diagram will be as follows

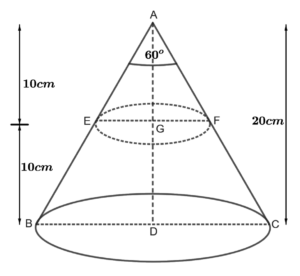
To get the values of radii of both ends of the frustum formed, compare ΔADB and ΔADC
Therefore, Volume of the wire = Volume of frustum of the cone
We will find the volume of the frustum by using formula;
Volume of frustum of a cone = 1/3 πh (r₁² + r₂² + r₁r₂), where r₁, r_2, and h are the radii and height of the frustum of the cone respectively. 
We will find the volume of the wire by using formula;
Volume of cylinder = πr²h, where r and h are radius and height of the cylinder
In ΔABC , EF parallel to BC and
AD = 20 cm
AG = 10 cm
∠BAC = 60°
To get the values of radii of both ends of the frustum formed compare ADB and ADC
AD = AD (common)
AB = AC (Slant height )
∠ADB = ∠ADC = 90 (Right circular cone)
ΔADB ≅ ΔADC (RHS criterion of congruency)
∠BAD = ∠DAC (CPCT)
Then,
∠BAD = ∠DAC 
∠BAD = 1/2 × 60° = 30°
In ΔADB
BD/AD = tan 30°
BD = AD tan 30°
BD = 20 cm × 1/√3
BD = 20√3/3 cm

In ΔAEG
EG/AG = tan 30°
EG = AG tan 30°
EG = 10 cm × 1/√3
EG = 10√3/3 cm

Height of the frustum of the cone, h = 10 cm
Radius of lower end, r₁ = (20√3) / 3 cm
Radius of upper end, r₂ = (10√3) / 3 cm
Diameter of the cylindrical wire, d = 1 / 16 cm
Radius of the cylindrical wire, r = 1 / 2 × 1 / 16 cm = 1 / 32 cm
Let the length of the wire be H
Since the frustum is drawn into wire
Volume of the cylindrical wire = Volume of frustum of the cone
πr²H = 1/3 πh (r₁² + r₂² + r₁r₂)
H = [h(r₁² + r₂² + r₁r₂)]/ 3r²
= [ 10 × {(20√3) /3)² + (10√3/ 3)² + (20√3) /3 × (10√3) /3] / 3 × (1/32)²
= [10 × {400/3 + 100/3  + 200/3}] / 3 × (1/1024)
= 10240 × 700 / 9
= 7168000 / 9
= 796444.44 cm
= 7964.4 m
Thus the length of the wire is 7964.4 m.

Go Back To Chapters