NCERT Solutions Class 10 Maths
Chapter – 12 (Areas Related to Circles)
The NCERT Solutions in English Language for Class 10 Mathematics Chapter – 12 Areas Related to Circles Exercise 12.3 has been provided here to help the students in solving the questions from this exercise.
Chapter : 12 Areas Related to Circles
Exercise – 12.3
1. Find the area of the shaded region in Fig. 12.19, if PQ = 24 cm, PR = 7 cm and O is the centre of the circle.
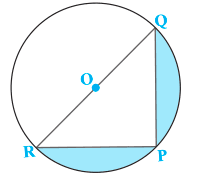
Solution – We know that, the angle in a semicircle is a right angle.
∴ ∠RPQ = 90°
Thus, ΔRQP is a right-angled triangle.
Given, PQ = 24 cm,
PR = 7 cm
∴ Using Pythagoras theorem,
RQ2 = PR2 + PQ2
RQ = √7² + 24²
= √49 + 576
= √625
Thus, PQ = 25 cm which is the diameter
∴ Radius (r) = 25/2 cm
Area of shaded region = Area of semicircle RPQ – Area of ΔRQP
= 1/2 × πr2 – 1/2 × PQ × RP
= 1/2 [(22/7 × 25/2 × 25/2) – (24 × 7)]
= 1/2 [6875/14 – 168]
= 1/2 [(6875 – 2352)/14]
= 1/2 × 4523/14
= 4523/28 cm2
= 161.54 cm2 (approximately)
2. Find the area of the shaded region in Fig. 12.20, if the radii of the two concentric circles with centre O are 7 cm and 14 cm, respectively and AOC = 40°.
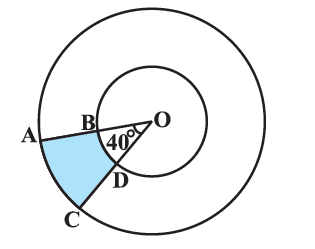
Solution – Given,
Angle made by sector = 40°,
Radius the inner circle = r = 7 cm, and
Radius of the outer circle = R = 14 cm
Area of shaded region ABDC = Area of sector ACO – Area of sector BDO
= θ/360° × πR2 – θ/360° × πr2
= θ/360° π (R2 – r2)
= θ/360° π (R + r )(R – r)
= 40°/360° × 22/7 × (14 + 7) (14 – 7)
= 1/9 × 22/7 × 21 × 7
= (22 × 21 × 7)/(9 × 7)
= (22 × 7)/3
= 154/3 cm2
= 51.33 cm2
3. Find the area of the shaded region in Fig. 12.21, if ABCD is a square of side 14 cm and APD and BPC are semicircles.

Solution – From figure, it is clear that the diameter of both the semicircles = Side of the square = 14 cm
∴ Radius of each semicircle (r) = 14/2 = 7 cm
Semicircles APD and BPC are drawn using sides AD and BC respectively as their diameter.
∴ Diameter of each semicircle = 14 cm
Radius of each semicircle (r) = 14/2 = 7 cm
Area of shaded region = Area of square ABCD – (Area of semicircle APD + Area of semicircle BPC)
= (side)2 – (1/2πr2 + 1/2πr2)
= (14)2 – π × (7)2
= 196 cm2 – 22/7 × 7 cm × 7 cm
= 196 cm2 – 154 cm2
= 42 cm2
4. Find the area of the shaded region in Fig. 12.22, where a circular arc of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm as the centre.
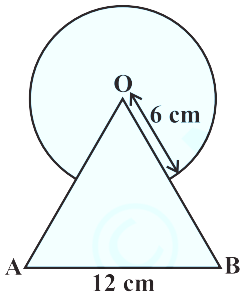
Solution – It is given that OAB is an equilateral triangle having each angle as 60°
The area of the sector is common in both.
Radius of circle (r) = 6 cm
Side of equilateral ΔOAB, (s) = 12 cm
We know each interior angle of equilateral Δ = 60°
∴ Area of shaded region = Area of circle + Area of ΔOAB – Area of sector OCDE
= πr2 + √3/4 (side)2 – θ/360° × πr2
= π(6 cm)2 + √3/4 (12)2 – 60°/360° × π (6 cm)2
= 36π cm² + 36√3 cm² – 6π cm²
= (30π + 36√3) cm²
= (30 × 22/7 + 36√3) cm²
= (36√3 + 660/7) cm²
5. From each corner of a square of side 4 cm, a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in Fig. 12.23. Find the area of the remaining portion of the square.

Solution –
Side of the square = 4 cm
The diameter of the circle which is cut out = 2 cm
∴ Radius of this circle (r) = 1 cm
Radius of all quadrants cut out (r) = 1 cm
Since all quadrants cut out are of the same radius thus,
Area of portions cut out of square = Area of the circle + 4 × (Area of each quadrant)
= πr2 + 4 (90°/360° × πr2)
= πr2 + 4 × πr2/4
= πr2 + πr2
= 2πr²
= 2π
= 2 × 22/7 cm2 =
= 44/7 cm2
Area of the remaining portion of the square = Area of square – Area of portion cut out
= (4 cm)2 – 44/7 cm2
= 16 cm2 – 44/7 cm2
= (112 – 44)/7 cm2
= 68/7 cm2
6. In a circular table cover of radius 32 cm, a design is formed, leaving an equilateral triangle ABC in the middle, as shown in Fig. 12.24. Find the area of the design.

Solution – Mark O as center of the circle. Join BO and CO.

Since we know that equal chords of a circle subtend equal angles at the center and all sides of an equilateral triangle are equal,
∴ Each side of triangle ABC will subtend equal angles at the center.
∴ ∠BOC = 360°/3 = 120°
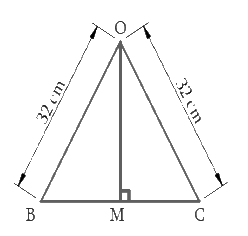
Consider ΔBOC. Drop a perpendicular from OM to BC
We know perpendicular from the center of circle to a chord bisects it.
⇒ BM = MC
OB = OC (radii)
OM = OM (common)
∴ ΔOBM ≅ ΔOCM (by SSS congruency)
⇒ ∠BOM = ∠COM (by CPCT)
∴ 2∠BOM = ∠BOC = 120°
∠BOM = 120°/2 = 60°
sin 60° = BM/BO = √3/2
∴ BM = √3/2 × BO = √3/2 × 32 = 16√3
⇒ BC = 2BM = 32√3
Using the formula of area of equilateral triangle = √3/4 (side)2
We can find the area of ΔABC since a side BC of ΔABC is known.
Visually from the figure, it’s clear that
Area of the design = Area of circle – Area of ΔABC = πr² – √3/4 (BC)²
This can be solved with ease as both the radius of the circle and BC are known.
Radius of circle (r) = 32 cm
From figure, we observe area of design = Area of circle – Area of ΔABC
= πr² – √3/4 (BC)²
= 22/7 × (32)² – √3/4 × (32√3)²
= 22/7 × 1024 – √3/4 × 1024 × 3
= 22528/7 – 768√3
Area of design = (22528/7 – 768√3) cm²
7. In Fig. 12.25, ABCD is a square of side 14 cm. With centres A, B, C and D, four circles are drawn such that each circle touch externally two of the remaining three circles. Find the area of the shaded region.
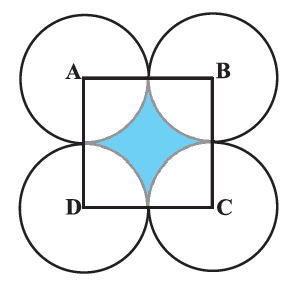
Solution – Side of square = 14 cm
Four quadrants are included in the four sides of the square.
∴ radius of the circles = 14/2 cm = 7 cm
Area of the square ABCD = 142 = 196 cm2
Area of the quadrant = (πR2)/4 cm2 = (22/7) ×72/4 cm2
= 77/2 cm2
Total area of the quadrant = 4 × 77/2 cm2 = 154cm2
Area of the shaded region = Area of the square ABCD – Area of the quadrant
= 196 cm2 – 154 cm2
= 42 cm2
8. Fig. 12.26 depicts a racing track whose left and right ends are semicircular.
 The distance between the two inner parallel line segments is 60 m and they are each 106 m long. If the track is 10 m wide, find
The distance between the two inner parallel line segments is 60 m and they are each 106 m long. If the track is 10 m wide, find
(i) the distance around the track along its inner edge
(ii) the area of the track.
Solution –
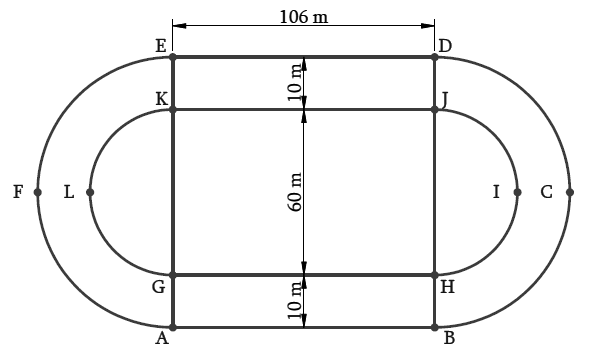
Given –
Width of track = 10 m
Radii of the inner semicircles HIJ and KLG (r₁) = 60/2 = 30 m
Radii of the outer semicircles BCD and EFA = 30 m + 10 m = 40 m
JK = GH = 106 m
DJ = HB = 10 m
(i) The distance around the track along its inner edge.
= GH + arc HIJ + JK + arc KLG
= 106 + 2πr₁/2 + 106 + 2πr₁/2
= 106 + π × 30 + 106 + π × 30
= 212 + 1320/7
= (1484 + 1320)/7
= 2804/7 m
(ii) Radius of semicircle BCD = Radius of semicircle EFA
(r₂) = 30 m + 10 = 40 m
Area of the track = Area of rectangle ABHG + Area of rectange KJDE + (Area of semicircle BCD – Area of semicircle HIJ) + (Area of semicircle EFA – Area of semicircle KLG)
= {(106 × 10) + (106 × 10) + [1/2π (40)² – 1/2π (30)²] + [1/2π (40)² – 1/2π (30)²]}
= {1060 + 1060 + [1/2π (1600 – 900)] + [1/2π (1600 – 900)] }
= 1060 + 1060 + π/2 × 700 + π/2 × 700
= 2120 + 700π
= 2120 + 700 × 22/7
= 2120 + 2200
= 4320 m2
9. In Fig. 12.27, AB and CD are two diameters of a circle (with centre O) perpendicular to each other, and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region.
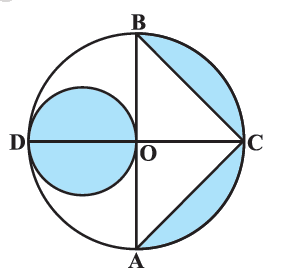
Solution – AB and CD are diameters of the circle with center O
∴ OD = OC = OA = OB = Radius of the circle R = 7 cm
∴ AB = 2R = 14 cm
Radius of shaded circular region, r = OD/2 = 7/2 cm
Area of the shaded smaller circular region = πr²
= π (7/2 cm)2
= 22/7 × 7/2 × 7/2 cm2
= 77/2 cm2
= 38.5 cm2
Area of the shaded segment of larger circular region = Area of semicircle ACB – Area of ΔABC
= 1/2 π(OA)2 – 1/2 × AB × OC
= 1/2 πR2 – 1/2 × 2R × R
= 1/2 × 22/7 × 72 – 1/2 × 14 × 7
= 77 – 49
= 28 cm2
Area of the shaded region = Area of the shaded smaller circular region + Area of the shaded segment of larger circular region
= 38.5 cm2 + 28 cm2
= 66.5 cm2
10. The area of an equilateral triangle ABC is 17320.5 cm2. With each vertex of the triangle as the centre, a circle is drawn with a radius equal to half the length of the side of the triangle (see Fig. 12.28). Find the area of the shaded region (Use π = 3.14 and √3 = 1.73205).
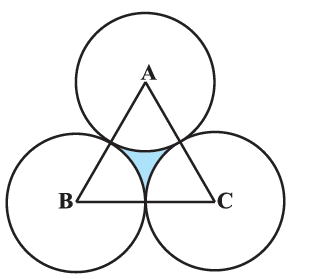
Solution – ABC is an equilateral triangle.
∴ ∠ A = ∠ B = ∠ C = 60°
There are three sectors, each making 60°.
Area of ΔABC = 17320.5 cm2
⇒ √3/4 × (side)2 = 17320.5
⇒ (side)2 =17320.5 × 4/1.73205
⇒ (side)2 = 4 × 104
⇒ side = 200 cm
Radius of the circles = 200/2 cm = 100 cm
Area of the sector = (60°/360°)×π r2 cm2
= 1/6 × 3.14 × (100)2 cm2
= 15700/3cm2
Area of 3 sectors = 3×15700/3 = 15700 cm2
Thus, the area of the shaded region = Area of an equilateral triangle ABC – Area of 3 sectors
= 17320.5-15700 cm2 = 1620.5 cm2
11. On a square handkerchief, nine circular designs, each of a radius 7 cm are made (see Fig. 12.29). Find the area of the remaining portion of the handkerchief.
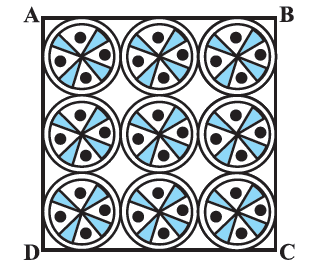
Solution – Number of circular designs = 9
The radius of the circular design = 7 cm
There are three circles on one side of the square handkerchief.
∴ side of the square = 3 × diameter of circle
= 3 × 14
= 42 cm
Area of the square = 42 × 42 cm2
= 1764 cm2
Area of the circle = πr2
= (22/7) × 7 × 7
= 154 cm2
Total area of the design = 9 × 154
= 1386 cm2
Area of the remaining portion of the handkerchief = Area of the square – Total area of the design
= 1764 – 1386
= 378 cm2
12. In Fig. 12.30, OACB is a quadrant of a circle with centre O and a radius 3.5 cm. If OD = 2 cm, find the area of the
(i) quadrant OACB
(ii) shaded region
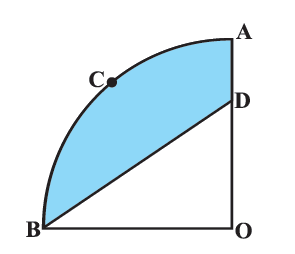
Solution – Radius of the quadrant = 3.5 cm = 7/2 cm
(i) Area of the quadrant OACB = (πR2)/4 cm2
= (22/7) × (7/2) × (7/2)/4 cm2
= 77/8 cm2
(ii) Area of the triangle BOD = (½) × (7/2) × 2 cm2
= 7/2 cm2
Area of the shaded region = Area of the quadrant – Area of the triangle BOD
= (77/8) – (7/2) cm2
= 49/8 cm2
= 6.125 cm2
13. In Fig. 12.31, a square OABC is inscribed in a quadrant OPBQ. If OA = 20 cm, find the area of the shaded region. (Use π = 3.14)

Solution – Side of square = OA = AB = 20 cm
The radius of the quadrant = OB
OAB is the right-angled triangle
By Pythagoras’ theorem in ΔOAB,
OB2 = AB2+OA2
⇒ OB2 = 202 +202
⇒ OB2 = 400 + 400
⇒ OB2 = 800
⇒ OB = 20√2 cm
Area of the quadrant = (πR2)/4 cm2
= (3.14/4)×(20√2)2 cm2
= 628cm2
Area of the square = 20 × 20 = 400 cm2
Area of the shaded region = Area of the quadrant – Area of the square
= 628-400 cm2 = 228cm2
14. AB and CD are, respectively, arcs of two concentric circles of radii 21 cm and 7 cm and centre O (see Fig. 12.32). If ∠AOB = 30°, find the area of the shaded region.

Solution –
The radius of the larger circle, R = 21 cm
The radius of the smaller circle, r = 7 cm
Angle made by sectors of both concentric circles = 30°
Area of the larger sector = (30°/360°) × πR2 cm2
= (1/12) × (22/7) × 212 cm2
= 231/2cm2
Area of the smaller circle = (30°/360°)×πr2 cm2
= 1/12 × 22/7 × 72 cm2
= 77/6 cm2
Area of the shaded region = (231/2) – (77/6) cm2
= 616/6 cm2
= 308/3cm2
15. In Fig. 12.33, ABC is a quadrant of a circle of radius 14 cm, and a semicircle is drawn with BC as a diameter. Find the area of the shaded region.

Solution – The radius of the quadrant ABC of the circle = 14 cm
AB = AC = 14 cm
BC is the diameter of the semicircle.
ABC is the right-angled triangle.
By Pythagoras’ theorem in ΔABC,
BC2 = AB2 +AC2
⇒ BC2 = 142 +142
⇒ BC = 14√2 cm
Radius of the semicircle = 14√2/2 cm = 7√2 cm
Area of the ΔABC =( ½) × 14 × 14 = 98 cm2
Area of the quadrant = (¼) × (22/7) × (14 × 14) = 154 cm2
Area of the semicircle = (½) × (22/7) × 7√2 × 7√2 = 154 cm2
Area of the shaded region =Area of the semicircle + Area of the ΔABC – Area of the quadrant
= 154 + 98 – 154 cm2
= 98cm2
16. Calculate the area of the designed region in Fig. 12.34 common between the two quadrants of circles of radius 8 cm each.
Solution –

AB = BC = CD = AD = 8 cm
Area of ΔABC = Area of ΔADC = (½) × 8 × 8 = 32 cm2
Area of quadrant AECB = Area of quadrant AFCD = (¼) × 22/7 × 82
= 352/7 cm2
Area of shaded region = (Area of quadrant AECB – Area of ΔABC)
= (Area of quadrant AFCD – Area of ΔADC)
= (352/7 – 32) + (352/7 – 32) cm2
= 2 × (352/7 – 32) cm2
= 256/7 cm2
Go Back To Chapters

