NCERT Solutions Class 9 Maths
Chapter – 7 (Triangles)
The NCERT Solutions in English Language for Class 9 Mathematics Chapter – 7 Triangles Exercise 7.4 has been provided here to help the students in solving the questions from this exercise.
Chapter 7: Triangles
- NCERT Solution Class 9 Maths Ex – 7.1
- NCERT Solution Class 9 Maths Ex – 7.2
- NCERT Solution Class 9 Maths Ex – 7.3
- NCERT Solution Class 9 Maths Ex – 7.5
Exercise – 7.4
1. Show that in a right-angled triangle, the hypotenuse is the longest side.
Answer – Let us consider a right-angled triangle ABC, right-angled at B.

In ∆ABC,
∠A + ∠B + ∠C = 180° (Angle sum property of a triangle)
∠A + 90o + ∠C = 180°
∠A + ∠C = 90°
Hence, the other two angles have to be acute (i.e., less than 90o ).
Thus, ∠B is the largest angle in ∆ABC.
So, ∠B > ∠A and ∠B > ∠C
Therefore, AC > BC and AC > AB [Using theorem 7.7 of triangles, in any triangle, the side opposite to the larger (greater) angle is longer.]
Therefore, AC is the largest side in ∆ABC.
However, AC is the hypotenuse of ∆ABC.
Therefore, the hypotenuse is the longest side in a right-angled triangle.
2. In Fig. 7.48, sides AB and AC of ΔABC are extended to points P and Q, respectively. Also, ∠PBC < ∠QCB. Show that AC > AB.
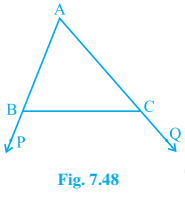
Answer – In the given figure,
∠ABC + ∠PBC = 180° [Linear pair of angles]
Also, ∠ABC = 180° – ∠PBC —————- (1)
∠ACB + ∠QCB = 180° [Linear pair]
∠ACB = 180° – ∠QCB —————- (2)
As ∠PBC < ∠QCB (given),
180o – ∠PBC > 180o – ∠QCB
∠ABC > ∠ACB [From Equations (1) and (2)]
Thus, AC > AB (Side opposite to the larger angle is larger).
Hence proved, AC > AB.
3. In Fig. 7.49, ∠B < ∠A and ∠C < ∠D. Show that AD < BC.
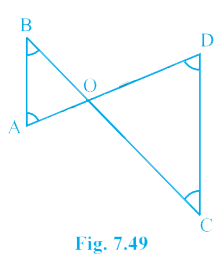
Answer – Given: ∠B < ∠A and ∠C < ∠D
To prove: AD < BC
We can use the fact that in any triangle, the side opposite to the larger (greater) angle is longer.
In ΔAOB, ∠B < ∠A (given)
AO < OB (The side opposite to the smaller angle is smaller) ————— (1)
In ΔCOD, ∠C < ∠D
OD < OC (The side opposite to the smaller angle is smaller) ————— (2)
On adding Equations (1) and (2), we obtain
AO + OD < BO + OC
AD < BC
Hence, proved
4. AB and CD are respectively the smallest and longest sides of a quadrilateral ABCD (see Fig. 7.50). Show that ∠A > ∠C and ∠B > ∠D.
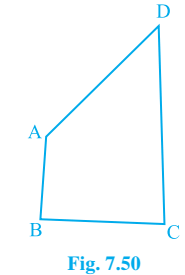
Answer – Given: AB and CD are respectively the smallest and longest sides of a quadrilateral ABCD
To prove: ∠A > ∠C and ∠B > ∠D
Let’s join vertex A to C and D to B as shown below.

In ΔABD, we see that
AB < AD < BD
So, ∠ADB < ∠ABD ———— (i) (Since the angle opposite to the longer side is always larger)
Now, in ΔBCD,
BC < DC < BD
Hence, it can be concluded that
∠BDC < ∠CBD ———— (ii)
Now, by adding equation (i) and equation (ii) we get,
∠ADB + ∠BDC < ∠ABD + ∠CBD
∠ADC < ∠ABC
∠B > ∠D
Similarly, In triangle ABC,
∠ACB < ∠BAC ———— (iii) (Since the angle opposite to the longer side is always larger)
Now, In ΔADC,
∠DCA < ∠DAC ———— (iv)
By adding equation (iii) and equation (iv), we get,
∠ACB + ∠DCA < ∠BAC+∠DAC
⇒ ∠BCD < ∠BAD
∴ ∠A > ∠C
5. In Fig 7.51, PR > PQ and PS bisect ∠QPR. Prove that ∠PSR > ∠PSQ.
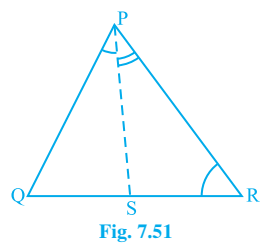
Answer – Given: PR > PQ and PS bisects ∠QPR
To prove: ∠PSR > ∠PSQ
As PR > PQ, ∠PQS > ∠PRS (Angle opposite to larger side is larger ) ———— (1)
PS is the angle bisector of ∠QPR.
∠QPS = ∠RPS ———— (2) [Since,PS bisects ∠QPR]
∠PSR is the exterior angle of ΔPQS.
∠PSR = ∠PQS + ∠QPS ———— (3) [Using exterior angle sum property]
∠PSQ is the exterior angle of ΔPRS.
∠PSQ = ∠PRS + ∠RPS ———— (4) [Using exterior angle sum property]
From Equations (1) and (2), we obtain
∠PQS + ∠QPS > ∠PRS + ∠RPS
∠PSR > ∠PSQ [From Equations (3) and (4)]
6. Show that of all line segments drawn from a given point not on it, the perpendicular line segment is the shortest.
Answer – We know that in a triangle if one angle is 90 degrees, then the other angles have to be acute.
Let us take a line l and from point P, that is, not on line l, draw two line segments PN and PM. Let PN be perpendicular to line l and PM is drawn at some other angle.
In ΔPNM, ∠N = 90o
∠P + ∠N + ∠M = 180o (Angle sum property of a triangle)
∠P + ∠M = 90o
Clearly, ∠M is an acute angle.
∠M < ∠N
PN < PM (The side opposite to the smaller angle is smaller)
Similarly, by drawing different line segments from P to l, it can be proved that PN is smaller in comparison to all of them. Therefore, it can be observed that of all line segments drawn from a given point not on it, the perpendicular line segment is the shortest.

