NCERT Solutions Class 9 Maths
Chapter – 7 (Triangles)
The NCERT Solutions in English Language for Class 9 Mathematics Chapter – 7 Triangles Exercise 7.2 has been provided here to help the students in solving the questions from this exercise.
Chapter 7: Triangles
- NCERT Solution Class 9 Maths Ex – 7.1
- NCERT Solution Class 9 Maths Ex – 7.3
- NCERT Solution Class 9 Maths Ex – 7.4
- NCERT Solution Class 9 Maths Ex – 7.5
Exercise – 7.2
1. In an isosceles triangle ABC, with AB = AC, the bisectors of ∠B and ∠C intersect each other at O. Join A to O. Show that:
(i) OB = OC
(ii) AO bisects ∠A
Answer – Given: AB = AC and the bisectors of ∠B and ∠C intersect each other at O.
Let’s construct a diagram according to the given question.
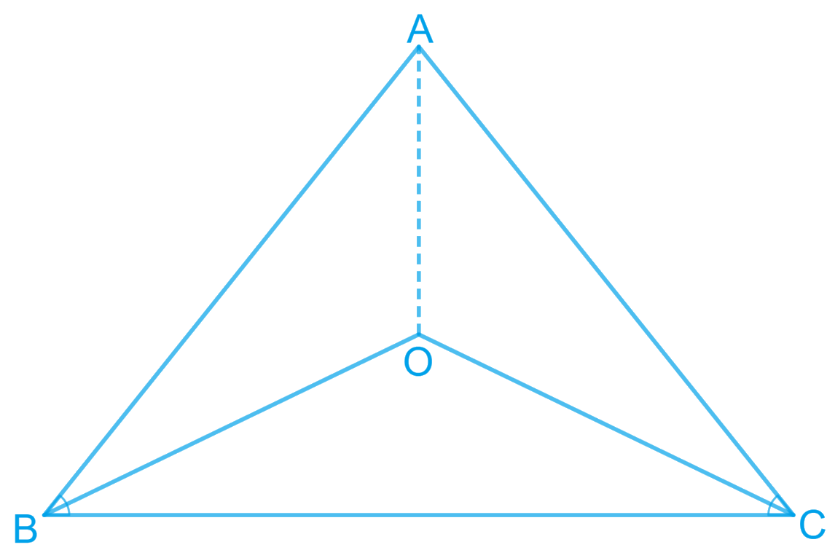
(i) OB = OC
It is given that in triangle ABC,
AB = AC (given)
∠ACB = ∠ABC (Angles opposite to equal sides of an isosceles triangle are equal)
∠ACB =
∠ABC
⇒ ∠OCB = ∠OBC (Since OB and OC are the angle bisectors of ∠ABC and ∠ACB)
∴ OB = OC (Sides opposite to equal angles of an isosceles triangle are
(ii) AO bisects ∠A
In ΔOAB and ΔOAC,
AO = AO (Common)
AB = AC (Given)
OB = OC (Proved above)
Therefore,
ΔOAB ≅ ΔOAC (By SSS congruence rule)
Also, we can use an alternative approach as shown below,
∠OBA = ∠OCA (OB and OC bisects angle ∠B and ∠C)
AB = AC (Given)
OB = OC (Proved above)
ΔOAB ≅ ΔOAC (By SAS congruence rule)
⇒ ∠BAO = ∠CAO (CPCT)
∴ AO bisects ∠A or AO is the angle bisector of ∠A.
2. In ΔABC, AD is the perpendicular bisector of BC (see Fig. 7.30). Show that ΔABC is an isosceles triangle in which AB = AC.
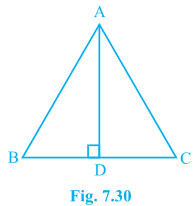
Answer – Given: AD is the perpendicular bisector of BC means ∠ADB = ∠ADC = 90° and BD = DC
To Prove: ΔABC is an isosceles triangle in which AB = AC.
In ΔADC and ΔADB,
AD = AD (Common)
∠ADC = ∠ADB (Each 90°)
CD = BD (AD is the perpendicular bisector of BC)
∴ ΔADC ≅ ΔADB (By SAS congruence rule)
∴ AB = AC (By CPCT)
Therefore, ABC is an isosceles triangle in which AB = AC.
3. ABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB, respectively (see Fig. 7.31). Show that these altitudes are equal.
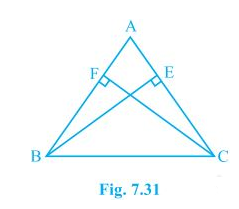
Answer – Given: ΔABC is an isosceles triangle
To prove: BE = CF
In ΔAEB and ΔAFC,
∠AEB = ∠AFC (Each 90° as BE and CF are altitudes)
∠A = ∠A (Common angle)
AB = AC (Given ΔABC is an isosceles triangle)
∴ ΔAEB ≅ ΔAFC (By AAS congruence rule)
∴ BE = CF (By CPCT)
4. ABC is a triangle in which altitudes BE and CF to sides AC and AB are equal (see Fig. 7.32). Show that
(i) ΔABE ≅ ΔACF
(ii) AB = AC, i.e. ABC is an isosceles triangle.
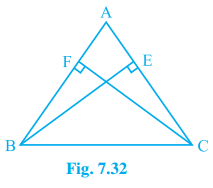
Answer – Given: BE = CF
To prove: (i) ΔABE ≅ ΔACF
In ΔABE and ΔACF,
∠AEB = ∠AFC (Each 90°)
∠A = ∠A (Common angle)
BE = CF (Given)
∴ ΔABE ≅ ΔACF (By AAS congruence rule)
To prove: (ii) AB = AC, i.e. ABC is an isosceles triangle.
We have proved above that ΔABE ≅ ΔACF
∴ AB = AC (By CPCT)
Hence, ΔABC is an isosceles triangle.
5. ABC and DBC are two isosceles triangles on the same base BC (see Fig. 7.33). Show that ∠ABD = ∠ACD.
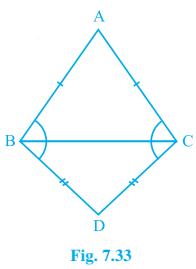
Answer – Given: ABC and DBC are isosceles triangles
To Prove: ∠ABD = ∠ACD
Let’s join point A and point B.
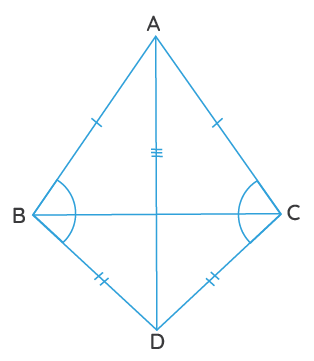
In △DAB and △DAC,
AB = AC (Given)
BD = CD (Given)
AD = AD (Common side)
∴ △ ABD ≅ △ ACD (By SSS congruence rule)
∴ ∠ABD = ∠ACD (By CPCT)
6. ΔABC is an isosceles triangle in which AB = AC. Side BA is produced to D such that AD = AB (see Fig. 7.34). Show that ∠BCD is a right angle.
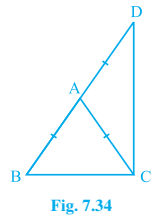
Answer – Given : AB = AC and AD = AB
To Prove: ∠BCD is a right angle.
In isosceles triangle ABC,
AB = AC (Given)
∴ ∠ACB = ∠ABC (Angles opposite to equal sides of a triangle are equal)
Let ∠ACB = ∠ABC be x. ———– (1)
In ΔACD,
AC = AD (Since, AB = AD)
∴ ∠ADC = ∠ACD (Angles opposite to equal sides of a triangle are equal)
Let ∠ADC = ∠ACD be y. ———– (2)
Thus,
∠BCD = ∠ACB + ∠ACD = x + y ———– (3)
In ΔBCD,
∠ABC + ∠BCD + ∠ADC = 180° (Angle sum property of a triangle)
Substituting the values we get,
x + (x + y) + y = 180° [From equation (1), (2) and (3)]
2 (x + y) = 180°
2(∠BCD) = 180° [From equation(3)]
∴ ∠BCD = 90°
7. ABC is a right-angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
Answer –
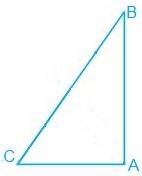
Given: ∠A = 90° and AB = AC
∴ ∠C = ∠B (Angles opposite to equal sides are also equal)
Let ∠B = ∠C = x
In ΔABC,
∠A + ∠B + ∠C = 180° (Angle sum property of a triangle)
90°+ x + x = 180°
90°+ 2x = 180°
2x = 90°
x = 45°
∴ ∠B = ∠C = 45°
8. Show that the angles of an equilateral triangle are 60° each.
Answer – Let ABC be an equilateral triangle, as shown below:

AB = BC = AC
∴ ∠C = ∠A = ∠B (Angles opposite to equal sides of a triangle are equal)
Let ∠A = ∠B = ∠C be x.
In △ ABC,
∠A + ∠B + ∠C = 180° (Angle sum property of a triangle)
⇒ x + x + x = 180°
⇒ 3x = 180°
⇒ x = 60°
∴ ∠A = ∠B = ∠C = 60°
Hence, in an equilateral triangle, all interior angles are of measure 60°.

