NCERT Solutions Class 9 Maths
Chapter – 7 (Triangles)
The NCERT Solutions in English Language for Class 9 Mathematics Chapter – 7 Triangles Exercise 7.1 has been provided here to help the students in solving the questions from this exercise.
Chapter 7: Triangles
- NCERT Solution Class 9 Maths Ex – 7.2
- NCERT Solution Class 9 Maths Ex – 7.3
- NCERT Solution Class 9 Maths Ex – 7.4
- NCERT Solution Class 9 Maths Ex – 7.5
Exercise – 7.1
1. In quadrilateral ACBD, AC = AD and AB bisect ∠A (see Fig. 7.16). Show that ΔABC≅ ΔABD. What can you say about BC and BD?
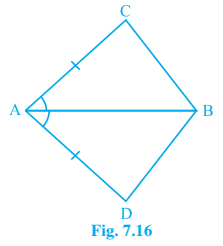
Answer – Given: AC = AD and AB bisects ∠A
To Prove: Δ ABC ≅ Δ ABD
We can show two sides and included angle of ABC are equal to the corresponding sides and included angle of ABD.
In Δ ABC and Δ ABD,
AC = AD (Given)
∠CAB = ∠DAB (AB bisects ∠A)
AB = AB (Common)
∴ Δ ABC ≅ Δ ABD (By SAS congruence rule)
∴ BC = BD (By CPCT)
Therefore, BC and BD are of equal lengths.
2. ABCD is a quadrilateral in which AD = BC and ∠DAB = ∠CBA (see Fig. 7.17). Prove that
(i) ΔABD ≅ ΔBAC
(ii) BD = AC
(iii) ∠ABD = ∠BAC.
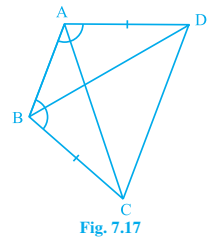
Answer – Given: AD = BC and ∠DAB = ∠CBA
To Prove: (i) △ ABD ≅ △ BAC
In △ABD and △BAC,
AD = BC (Given)
∠DAB = ∠CBA (Given)
AB = BA (Common)
∴ △ABD ≅ △BAC (By SAS congruence rule)
To Prove: (ii) BD = AC
Since △ABD ≅ △BAC,
∴ BD = AC (By CPCT)
To Prove: (iii) ∠ABD = ∠BAC
Since ΔABD ≅ ΔBAC so,
Angles ∠ABD = ∠BAC (by the rule of CPCT).
3. AD and BC are equal perpendiculars to a line segment AB (see Fig. 7.18). Show that CD bisects AB.
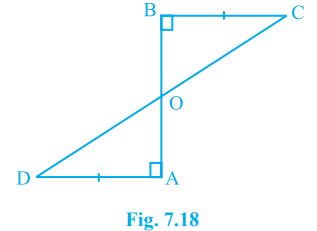
Answer – Given: AD ⊥ AB, BC ⊥ AB, and AD = BC
To Prove: CD bisects AB or OA = OB
We can show that the two triangles OBC and OAD are congruent by using AAS congruency rule and then we can say corresponding parts of congruent triangles will be equal.
Consider two triangles △ BOC and △ AOD,
In △ BOC and △ AOD,
∠BOC = ∠AOD (Vertically opposite angles)
∠CBO = ∠DAO (Each 90º, since AD and BC are ⊥ to AB)
BC = AD (Given)
∴ △BOC ≅ △AOD (AAS congruence rule)
∴ BO = AO (By CPCT)
Thus, CD bisects AB and O is the mid-point of AB.
4. l and m are two parallel lines intersected by another pair of parallel lines p and q (see Fig. 7.19). Show that ΔABC ≅ ΔCDA.
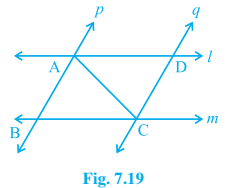
Answer – Given: l || m and p || q
To Prove: ΔABC ≅ ΔCDA.
We can show both the triangles are congruent by using ASA congruency criterion
In ΔABC and ΔCDA,
∠BAC = ∠DCA (Alternate interior angles, as p and q are parallel lines)
AC = CA (Common)
∠BCA = ∠DAC (Alternate interior angles, as l || m)
∴ ΔABC ≅ ΔCDA (By ASA congruence rule)
5. Line l is the bisector of an angle ∠A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠A (see Fig. 7.20). Show that:
(i) ΔAPB ≅ ΔAQB
(ii) BP = BQ or B is equidistant from the arms of ∠A.
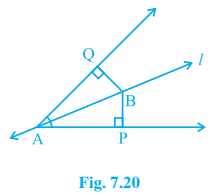
Answer – Given: l is the bisector of an angle ∠A and BP ⊥ AP and BQ ⊥ AQ
To Prove: ΔAPB ≅ ΔAQB and BP = BQ
(i) We can show two triangles APB and AQB are congruent by using AAS congruency rule and then show that the corresponding parts of congruent triangles will be equal.
In ΔAPB and ΔAQB,
∠BAP = ∠BAQ (l is the angle bisector of ∠A)
∠APB = ∠AQB (Each 90°)
AB = AB (Common)
∴ ΔAPB ≅ ΔAQB (By AAS congruence rule)
(ii) Since, ΔAPB ≅ ΔAQB
∴ BP = BQ (By CPCT)
Or, it can be said that point B is equidistant from the arms of ∠A.
6. In Fig. 7.21, AC = AE, AB = AD and ∠BAD = ∠EAC. Show that BC = DE.
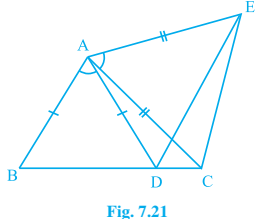
Answer – Given: AC = AE, AB = AD and ∠BAD = ∠EAC
To Prove: BC = DE
We can show two triangles BAC and DAE are congruent triangles by using SAS congruency rule and then we can say corresponding parts of congruent triangles will be equal.
It is given that ∠BAD = ∠EAC
Thus, by adding ∠DAC to both sides of this equation, we get
∠BAD + ∠DAC = ∠EAC + ∠DAC (∠DAC is common)
∠BAC = ∠DAE
In ΔBAC and ΔDAE,
AB = AD (Given)
∠BAC = ∠DAE (Proven above)
AC = AE (Given)
∴ ΔBAC ≅ ΔDAE (By SAS congruence rule)
∴ BC = DE (By CPCT)
7. AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠BAD = ∠ABE and ∠EPA = ∠DPB (see Fig. 7.22). Show that
(i) ΔDAP ≅ ΔEBP
(ii) AD = BE
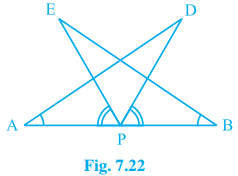
Answer – Given: P is its mid-point of AB, ∠BAD = ∠ABE and ∠EPA = ∠DPB
We can show two triangles DAP and EBP congruent by using ASA congruency rule and then we can say corresponding parts of congruent triangles will be equal.
To Prove: (i) ΔDAP ≅ ΔEBP
It is given that ∠EPA = ∠DPB
∠EPA + ∠DPE = ∠DPB + ∠DPE (∠DPE is common)
∴ ∠DPA = ∠EPB
In ΔDAP and ΔEBP,
∠DAP = ∠EBP (Given)
AP = BP (P is mid – point of AB)
∠DPA = ∠EPB (Proven above)
∴ ΔDAP ≅ ΔEBP (ASA congruence rule)
To Prove: (ii) AD = BE.
Since, ΔDAP ≅ ΔEBP
∴ AD = BE (By CPCT)
8. In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see Fig. 7.23). Show that:
(i) ΔAMC ≅ ΔBMD
(ii) ∠DBC is a right angle.
(iii) ΔDBC ≅ ΔACB
(iv) CM = AB
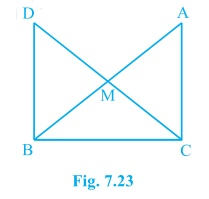
Answer – Given: M is the mid-point of hypotenuse AB, ∠C = 90° and DM = CM
To Prove: (i) ΔAMC ≅ ΔBMD
In ΔAMC and ΔBMD,
AM = BM (M is the mid – point of AB)
∠AMC = ∠BMD (Vertically opposite angles)
CM = DM (Given)
∴ ΔAMC ≅ ΔBMD (By SAS congruence rule)
∴ AC = BD (By CPCT)
Also, ∠ACM = ∠BDM (By CPCT)
To Prove: (ii) ∠DBC is a right angle.
∠DBC is a right angle.
We know that, ∠ACM = ∠BDM (proved above)
But, ∠ACM and ∠BDM are alternate interior angles. Since alternate angles are equal, it can be said that DB || AC.
∠DBC + ∠ACB = 180° (Co-interior angles)
∠DBC + 90° = 180° [Since, ΔACB is a right angled triangle]
∴ ∠DBC = 90°
Thus, ∠DBC is a right angle.
To Prove: (iii) ΔDBC ≅ ΔACB
In ΔDBC and ΔACB,
DB = AC (Already proved)
∠DBC = ∠ACB = 90° (Proved above)
BC = CB(Common)
∴ Δ DBC ≅ Δ ACB (SAS congruence rule)
To Prove: (iv) CM = AB
DC = AB (Since ΔDBC ≅ ΔACB)
⇒ DM = CM = AM = BM (Since M the is mid-point)
So, DM + CM = BM + AM
Hence, CM + CM = AB
⇒ CM = AB

