NCERT Solutions Class 9 Maths Chapter 13 Surface Areas and Volumes Ex 13.7

NCERT Solutions Class 9 Maths
Chapter – 13 (Surface Areas and Volumes)

The NCERT Solutions in English Language for Class 9 Mathematics Chapter – 13 Surface Areas and Volumes Exercise 13.7 has been provided here to help the students in solving the questions from this exercise.

Chapter 13: Surface Areas and Volumes

Exercise – 13.7

(Assume π = $\mathbf{\frac{22}{7}}$ , unless stated otherwise)

1. Find the volume of the right circular cone with
(i) radius 6 cm, height 7 cm
(ii) radius 3.5 cm, height 12 cm

Answer –
(i) Radius of the cone, r = 6 cm
Height of cone, h = 7 cm
Volume of the cone = $\frac{1}{3}$ πr²h
= $\frac{1}{3}$ × $\frac{22}{7}$ × 36 × 7
= 12 × 22
= 264
The volume of the cone is 264 cm³.

(ii) Radius of the cone, r = 3.5 cm
Height of the cone, h = 12 cm
Volume of the cone = $\frac{1}{3}$ πr²h
= $\frac{1}{3}$ × $\frac{22}{7}$ × 3.5²× 7
= 154
The volume of the cone is 154 cm³.

2. Find the capacity in litres of a conical vessel with
(i) radius 7cm, slant height 25 cm
(ii) height 12 cm, slant height 13 cm

Answer –
(i) Radius of the cone, r =7 cm
Slant height of the cone, l = 25 cm
Height of the conical vessel, h = $\sqrt{l^2 - r^2}$
= √(25)² – (7)²
= √625 – 49
= √576
h = 24 cm
Capacity of the conical vessel = $\frac{1}{3}$ πr²h
= $\frac{1}{3}$ × $\frac{22}{7}$ × 7 cm × 7 cm × 24 cm
= 1232 cm³
= 1232 × (1/1000L) [∵ 1000 cm³ = 1 litre]
= 1.232 litres

(ii) Height of the conical vessel, h = 7 cm
Slant height of the conical vessel, l = 13 cm

Radius of the conical vessel, r = $\sqrt{l^2 - h^2}$
= √(13)² – (12)²
= √169 -144
= √25
r = 5 cm
Capacity of the conical vessel = $\frac{1}{3}$ πr²h
= $\frac{1}{3}$ × $\frac{22}{7}$ × 5 cm × 5 cm × 12 cm
= 2200/7 cm³
= 2200/7 × 1/1000 l [∵ 1000 cm³ = 1 litre]
= 11/35 litres

3. The height of a cone is 15cm. If its volume is 1570cm³, find the diameter of its base. (Use π = 3.14)

Answer –
Let the radius of the cone = r
Height of the cone, h = 15 cm
Volume of the cone = 1570 cm³ $\frac{1}{3}$ πr²h = 1570

$\frac{1}{3}$ × 3.14 × r²× 15 = 1570
r² = $\frac{1570\times 3}{3.14\times 15}$
r² = 100
r = 10
Radius of the base of the cone is 10 cm.

4. If the volume of a right circular cone of height 9cm is 48πcm³, find the diameter of its base.

Answer –
Let the radius of the cone = r
Height of cone, h = 9 cm
Volume of cone = 48π cm³ $\frac{1}{3}$ πr²h = 48π

$\frac{1}{3}$ πr²× 9 = 48 π
r² = 16
r = 4
Radius of the cone is 4 cm.
So, diameter = 2×Radius = 8
Thus, diameter of the base is 8 cm.

5. A conical pit of top diameter 3.5m is 12m deep. What is its capacity in kiloliters?

Answer –
Diameter of the conical pit = 3.5 m
Radius of the conical pit, r = diameter/ 2 = (3.5/2)m = 1.75m
Height of the pit, h = Depth of the pit = 12m
Volume of the cone, V = $\frac{1}{3}$ πr²h
V = $\frac{1}{3}$ × $\frac{22}{7}$ × (1.75)²× 12
= 38.5
Volume of the cone is 38.5 m³Hence, capacity of the pit = (38.5 × 1) kiloliters = 38.5 kiloliters.

6. The volume of a right circular cone is 9856cm³. If the diameter of the base is 28cm, find
(i) height of the cone
(ii) slant height of the cone
(iii) curved surface area of the cone

Answer –
Diameter of the cone, d = 28 cm
Radius of the cone, r = 28/2 cm = 14 cm
Volume of a right circular cone = 9856 cm³

(i) Let the height of the cone be h
Volume of the cone, V = $\frac{1}{3}$ πr²h
$\frac{1}{3}$ πr²h = 9856

$\frac{1}{3}$ × $\frac{22}{7}$ × 14 × 14 × h = 9856
h = 48
The height of the cone is 48 cm.

(ii) Slant height of the cone = l
l = √r² + h²
= √(14)² + (48)²
= √(196 + 2304)
= √(2500)
= 50 cm
Slant height of the cone is 50 cm.

(iii) curved surface area of the cone = πrl
= $\frac{22}{7}$ × 14 × 50
= 2200
The curved surface area of the cone is 2200 cm².

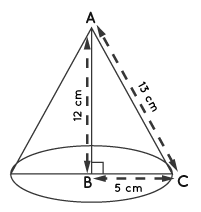
7. A right triangle ABC with sides 5cm, 12cm and 13cm is revolved about the side 12 cm. Find the volume of the solid so obtained.
Answer –
Height (h)= 12 cm
Radius (r) = 5 cm, and
Slant height (l) = 13 cm
NCERT Class 9 Solutions Maths
Volume of cone, V = $\frac{1}{3}$ πr²h
V = $\frac{1}{3}$ × π × 5²× 12
= 100π
The volume of the cone so formed is 100π cm³.

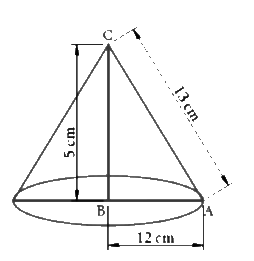
8. If the triangle ABC in the Question 7 is revolved about the side 5cm, then find the volume of the solids so obtained. Find also the ratio of the volumes of the two solids obtained in Questions 7 and 8.

Answer – Radius of the cone, r =12 cm
Height of the cone, h = 5 cm
NCERT Class 9 Solutions Maths

Volume of the cone = $\frac{1}{3}$ πr²h
= $\frac{1}{3}$ × π × 12cm × 12cm × 5cm
= 240π cm³
Volume of the cone in question 7 = 100π cm
Ratio = Volume of the cone in question 7 : Volume of the cone in question 8
= 100π : 240π
= 5 : 12
The volume of the cone is 240π cm³ and the required ratio is 5 : 12.

9. A heap of wheat is in the form of a cone whose diameter is 10.5 m and height is 3 m. Find its volume. The heap is to be covered by canvas to protect it from rain. Find the area of the canvas.

Answer –
Diameter of the conical heap, d = 10.5 m
Radius of the conical heap, r = 10.5/2 m = 5.25 m
Height of the conical heap, h = 3 m
Volume of heap = $\frac{1}{3}$ πr²h
= $\frac{1}{3}$ × $\frac{22}{7}$ × 5.25 × 5.25 × 3
= 86.625
The volume of the heap of wheat is 86.625 m³.
Slant height, l = √r² + h²
= √(5.25)² + (3)²
= √(27.5625 + 9)
= √(36.5625)
= 6.046 m (approx.)
The area of the canvas required to cover the heap of wheat = πrl
= $\frac{22}{7}$ × 5.25 m × 6.046 m
= 99.759 m²
The volume of the conical heap is 86.625 m³ and the area of the canvas required is 99.759 m².

NCERT Solutions Class 9 Maths Chapter 13 Surface Areas and Volumes Ex 13.7