NCERT Solutions Class 9 Maths
Chapter – 11 (Constructions)
The NCERT Solutions in English Language for Class 9 Mathematics Chapter – 11 Constructions Exercise 11.1 has been provided here to help the students in solving the questions from this exercise.
Chapter 11: Constructions
Exercise – 11.1
1. Construct an angle of 90° at the initial point of a given ray and justify the construction.
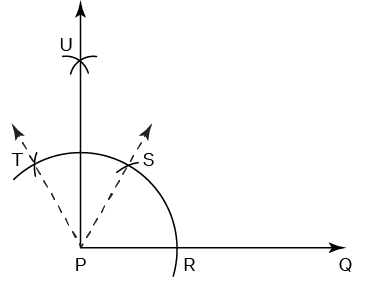
Answer – Steps of Construction :
1. Draw a ray PQ.
2. Take P as a centre with any radius, draw an arc TSR is that cuts PQ at R.
3. With R as a centre with the same radius, mark a point S on the arc TSR.
4. With S as a centre and the same radius, mark a point T on the arc TSR.
5. Take S and T as centre, draw two arcs which intersect each other with the same radius at U.
6. Finally, the ray PU is joined which makes an angle 90° with PU is formed.
Justification
To prove ∠UPQ = 90°
∠SPQ = 60°
∠TPS = 60°
∠UPS = ∠TPS = 30°
∠UPQ = ∠UPS + ∠SPR
∠UPQ = 30° + 60° = 90°
Hence, justified.
2. Construct an angle of 45° at the initial point of a given ray and justify the construction.
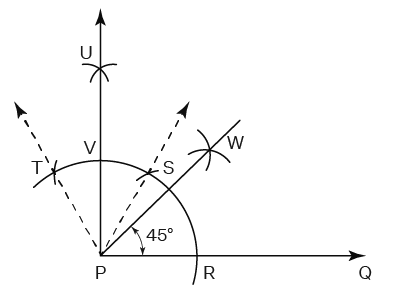
Answer – Steps of Construction :
1. Draw a ray PQ.
2. Take U as a centre with any radius, draw an arc TSR that cuts PQ at R.
3. With R as a centre with the same radius, mark a point S on the arc TSR.
4. With R as a centre and the same radius, mark a point T on the arc TSR.
5. Take S and T as centre, draw two arcs which intersect each other with the same radius at U.
6. Finally, the ray PU is joined which makes an angle 90° with PU is formed.
7. Take R and V as centre draw the perpendicular bisector which intersects at the point W.
8. Draw a line that joins the point P and W.
9. So, the angle formed ∠WPQ = 45°
Justification
∠UPQ = 90°
∠WPQ = ∠POA
∠WPQ = × 90° = 45°
Hence, justified.
3. Construct the angles of the following measurements:
(i) 30°
(ii)
(iii) 15°
Answer –
(i) 30°
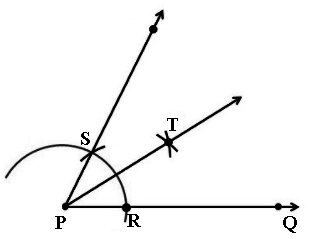
Steps of Construction :
1. Draw a ray PQ.
2. Take P as a centre with any radius, draw an arc RS which cuts PQ at R.
3. With R and S as centres, draw two arcs which intersect each other at the point T and the perpendicular bisector is drawn.
4. Thus, ∠TPQ is the required angle making 30° with PQ.

Steps of Construction :
1. Draw a ray PQ.
2. To construct an angle of 60°, with P as a center and any radius, draw a wide arc to intersect PQ at R. With R as a center and same radius draw an arc to intersect the initial arc at S. Then, ∠SPR = 60°
3. To construct an adjacent angle of 60°, with S as the center and the same radius as before, draw an arc to intersect the initial arc at T. Then, ∠TPS = 60°
4. To bisect ∠TPS, with T and S as centers and radius greater than half of TS , draw arcs to intersect each other at Z. Join P and Z. Then, ∠ZPQ = 90°e) To bisect ∠ZPQ, with R and U as centers and radius more than half of RU, draw arcs to intersect each other at V. Join P and V. Then, ∠VPQ = 45°
5. To bisect ∠VPQ = 45°, with W and R as centers and radius greater than half of WR, draw arcs to intersect each other at X. Join P and X. PX bisects ∠VPQ.
Hence, ∠XPQ = ∠WPQ =
× 45° = °
(iii) 15°
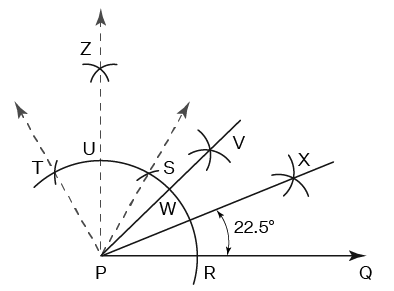
Steps of Construction :
1. Draw a ray PQ.
2. To construct an angle of 60°, with P as a center and any radius, draw a wide arc to intersect PQ at R. With R as a center and same radius draw an arc to intersect the initial arc at S. Then, ∠SPR = 60°
3. Now, we will bisect ∠SPR. With R and S as centers and radius greater than half of RS, draw arcs to intersect each other at T. Join P and T. So, PT is the angle bisector of ∠SPR.
∠TPQ = ∠SPR =
× 60° = 30°
4. To bisect ∠TPQ, With R and W as centers and radius greater than half of arc RW, draw arcs to intersect each other at U. Join P and U. PU is the angle bisector of ∠TPQ .
∠UPQ = ∠TPQ =
× 30° = 15°

4. Construct the following angles and verify by measuring them by a protractor:
(i) 75°
(ii) 105°
(iii) 135°
Answer –
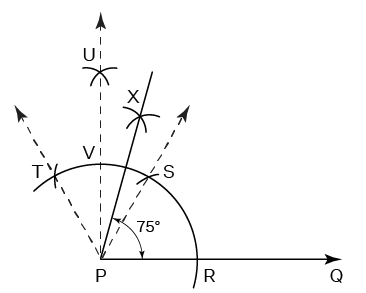
(i) 75°
Steps of Construction :
1. Draw a ray PQ.
2. To construct an angle of 60°, with P as a center and any radius, draw a wide arc to intersect PQ at R. With R as a center and same radius, draw an arc to intersect the initial arc at S. Thus,∠SPR = 60°
3. To construct an adjacent angle of 60° with S as a center and the same radius, draw an arc that intersects the initial arc at T.
4. To bisect ∠SPT, with T and S as centers and radius more than half of TS draw arcs to bisect each other at U. Join U and P.
∠UPS = ∠TPS =
× 60° = 30°
5. To bisect ∠UPS, with V and S as centers and radius greater than half of VS draw arcs to intersect each other at X.
∠XPS = ∠UPS =
× 30° = 15°
∠XPQ = ∠XPS + ∠SPQ
= 15° + 60° = 75°
Thus, ∠XPQ = 75°
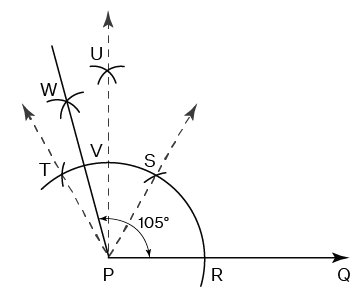
(ii) 105°
Steps of Construction :
1. Draw a ray PQ.
2. To construct an angle of 60° With P as a center and any radius, draw a wide arc to intersect PQ at R. With R as a center and same radius, draw an arc to intersect the initial arc at S. Thus, ∠SPR = 60°.
3. To construct an adjacent angle with S as the center and the same radius as before, draw an arc to intersect the initial arc at T. ∠TPS = 60°.
4. To bisect ∠TPS, with T and S as centers and radius greater than TS draw arcs to bisect each other at U. Join U and P.
∠UPS = ∠TPS =
× 60° = 30°
∠UPT = ∠UPS = 30°
5. To bisect ∠UPT, with T and V as centers and radius greater than half of TV, draw arcs to intersect each other at W. Join P and W.
∠WPU = ∠UPT =
× 30° = 15°
∠WPR = ∠WPU + ∠UPS + ∠SPR
= 15° + 30° + 60°
= 105°
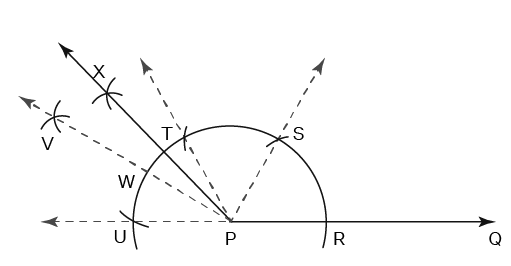
(iii) 135°
Steps of Construction :
1. Draw a ray PQ.
2. To construct an angle of 60°, with P as a center and any radius, draw a wide arc to intersect PQ at R. Thus, ∠SPR = 60°.
3. To construct an adjacent angle of 60°, with S as the center and the same radius as before, draw an arc to intersect the initial arc at T. Thus, ∠TPS = 60°.
4. To construct the second adjacent angle of 60°, with T as a center and the same radius as before, draw an arc to intersect the initial arc at U. Thus, ∠UPT = 60°
5. To bisect ∠UPT, with T and U as centers and radius greater than half of TU, draw two arcs to intersect each other at V.
∠VPT = ∠VPU = ∠UPT =
× 60° = 30°
6. To bisect ∠VPT, with W and T as centers and radius greater than half of WT, draw arcs to intersect each other at X.
∠XPT = ∠XPV = ∠VPT =
× 30° = 15°
∠XPQ = ∠XPT + ∠TPS + ∠SPR
= 15° + 60° + 60°
= 135°
Thus, ∠XPQ = 135°
5. Construct an equilateral triangle, given its side and justify the construction.
Answer – Steps of Construction:
1. Draw a ray AB.
2. With A as center and radius equal to 3 cm, draw an arc to cut ray AB at C such that AC = 3 cm.
3. With C as the center and radius equal to AC (3cm), draw an arc to intersect the initial arc at D.
4. Join AD and DC.
5. Triangle ADC is an equilateral triangle with sides of 3cm each.
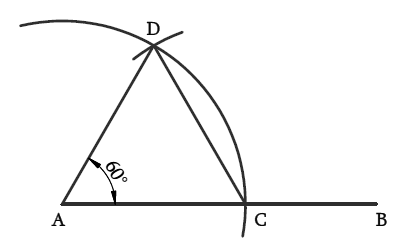
Justification:
AC = AD (By construction since the radius of the arc is the same)
AC = CD (By construction since the same radius was used again)
Hence AC = AD = CD = 3cm
Thus, ADC is an equilateral triangle.

