NCERT Solutions Class 9 Maths
Chapter – 10 (Circles)
The NCERT Solutions in English Language for Class 9 Mathematics Chapter – 10 Circles Exercise 10.5 has been provided here to help the students in solving the questions from this exercise.
Chapter 10: Circles
- NCERT Solution Class 9 Maths Ex – 10.1
- NCERT Solution Class 9 Maths Ex – 10.2
- NCERT Solution Class 9 Maths Ex – 10.3
- NCERT Solution Class 9 Maths Ex – 10.4
- NCERT Solution Class 9 Maths Ex – 10.6
Exercise – 10.5
1. In Fig. 10.36, A, B and C are three points on a circle with centre O, such that ∠BOC = 30° and ∠AOB = 60°. If D is a point on the circle other than the arc ABC, find ∠ADC.
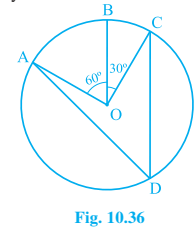
Answer – The angle subtended by an arc at the center is double the angle subtended by it at any point on the remaining part of the circle.
∠AOC = ∠AOB + ∠BOC = 90°
∠AOC = 2 ∠ADC (By Theorem 10.8)
∠ADC = ∠AOC
∠ADC = × 90 = 45°
∴ ∠ADC = 45°
2. A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.
Answer – The angle subtended by an arc at the center is double the angle subtended by it at any point on the remaining part of the circle.
A quadrilateral ABCD is called cyclic if all the four vertices of it lie on a circle and the sum of either pair of opposite angles of a cyclic quadrilateral is 180°.
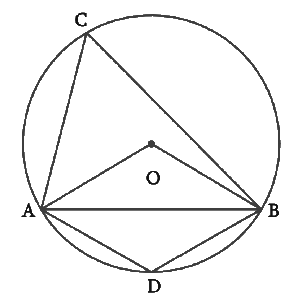
Here OA = OB = AB
Hence ∆ABO becomes an equilateral triangle.
Draw 2 points C and D on the circle such that they lie on the major arc and minor arc, respectively.
Since ∆ABO is an equilateral triangle, we get ∠AOB = 60°.
For the arc AB, ∠AOB = 2∠ACB as we know that the angle subtended by an arc at the center is double the angle subtended by it at any point on the remaining part of the circle.
∠ACB = ∠AOB =
× 60 = 30°
As you can notice the points A, B, C, and D lie on the circle. Hence ABCD is a cyclic quadrilateral.
We know that the sum of either pair of opposite angles of a cyclic quadrilateral is 180°.
Therefore,
∠ACB + ∠ADB = 180°
30° + ∠ADB = 180°
∠ADB = 150°
So, when the chord of a circle is equal to the radius of the circle, the angle subtended by the chord at a point on the minor arc is 150° and also at a point on the major arc is 30°.
3. In Fig. 10.37, ∠PQR = 100°, where P, Q and R are points on a circle with centre O. Find ∠OPR.
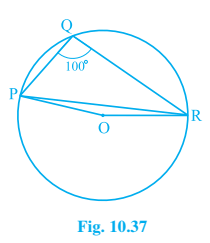
Answer – Since the angle which is subtended by an arc at the centre of the circle is double the angle subtended by that arc at any point on the remaining part of the circle.
So, the reflex ∠POR = 2 × ∠PQR
We know the values of angle PQR as 100°.
So, ∠POR = 2×100° = 200°
∴ ∠POR = 360° – 200° = 160°
Now, in ΔOPR,
OP and OR are the radii of the circle.
So, OP = OR
Also, ∠OPR = ∠ORP
Now, we know the sum of the angles in a triangle is equal to 180 degrees.
So,
∠POR + ∠OPR + ∠ORP = 180°
∠OPR + ∠OPR = 180° – 160°
As ∠OPR = ∠ORP
2 ∠OPR = 20°
Thus, ∠OPR = 10°
4. In Fig. 10.38, ∠ABC = 69°, ∠ACB = 31°, find ∠BDC.
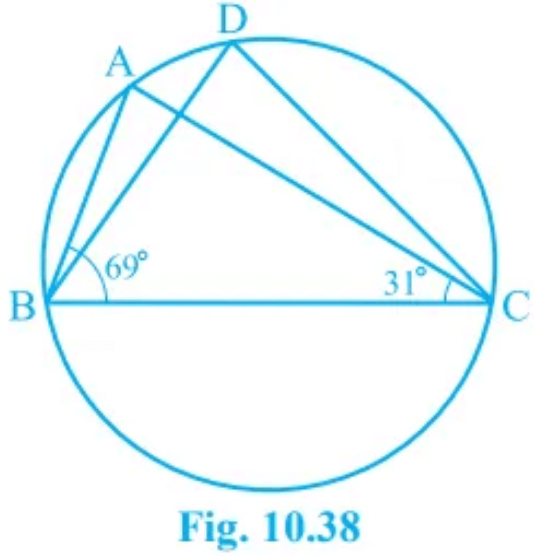
Answer – We know that angles in the segment of the circle are equal, so,
∠BAC = ∠BDC
Now, in the ΔABC, the sum of all the interior angles will be 180°.
So, ∠ABC + ∠BAC + ∠ACB = 180°
Now, by putting the values,
∠BAC = 180° – 69° – 31°
∴ ∠BDC = 80°
5. In Fig. 10.39, A, B, C and D are four points on a circle. AC and BD intersect at a point E, such that ∠ BEC = 130° and ∠ ECD = 20°. Find BAC.
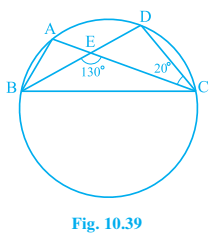
Answer – Consider the straight-line BD. As the line AC intersects with the line BD, the sum of two adjacent angles so formed is 180°.
Therefore, ∠BEC + ∠DEC = 180°
130° + ∠DEC = 180°
∠DEC = 180° – 130° = 50°
Consider the ∆DEC, the sum of all angles will be 180º.
∠DEC + ∠EDC + ∠ECD = 180°
50° + ∠EDC + 20° = 180°
∠EDC = 180° – 70° = 110°
∴ ∠BDC = ∠EDC = 110°
We know that angles in the same segment of a circle are equal.
∴ ∠BAC = ∠BDC = 110°
6. ABCD is a cyclic quadrilateral whose diagonals intersect at point E. If ∠ DBC = 70°, ∠ BAC is 30°, find ∠ BCD. Further, if AB = BC, find ∠ ECD.
Answer – Consider the following diagram.
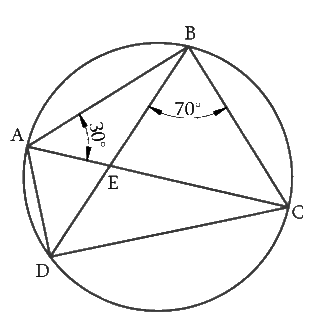
In the triangles, ABD and BCD, ∠CAD = ∠CBD = 70°. (Angles in the same segment are equal)
Hence, ∠BAD = ∠CAB + ∠DAC
= 30° + 70° = 100°
Thus, ∠BAD = 100°
Since ABCD is a cyclic quadrilateral, the sum of either pair of opposite angles of a cyclic quadrilateral is 180º.
∠BAD + ∠BCD = 180°
∠BCD = 180° – 100° = 80°
Thus, ∠BCD = 80°
Also given AB = BC.
So, ∠BCA = ∠BAC = 30° (Base angles of isosceles triangle are equal)
∠ECD = ∠BCD – ∠BCA
∠ECD = 80° – 30°
∠ECD = 50°
Thus, ∠ECD = 50°
7. If diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.
Answer – Draw a cyclic quadrilateral ABCD inside a circle with centre O, such that its diagonal AC and BD are two diameters of the circle.
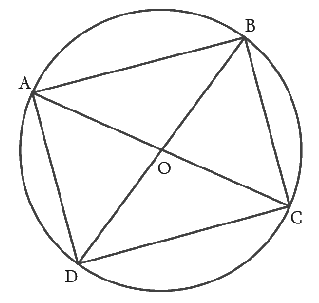
We know that the angles in the semi-circle are equal.
So, ∠ ABC = ∠ BCD = ∠ CDA = ∠ DAB = 90°
So, as each internal angle is 90°, it can be said that the quadrilateral ABCD is a rectangle.
8. If the non-parallel sides of a trapezium are equal, prove that it is cyclic.
Answer – We know that, if the sum of a pair of opposite angles of a quadrilateral is 180°, the quadrilateral is cyclic.
Draw a trapezium ABCD with AB || CD
AD and BC are the non-parallel sides that are equal. AD = BC. Draw AM ⊥ CD and BN ⊥ CD.
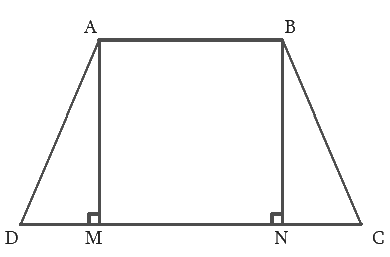
Consider ΔAMD and ΔBNC
AD = BC (Given)
∠AMD = ∠BNC (90°)
AM = BN (Perpendicular distance between two parallel lines is same)
By RHS congruence, ΔAMD ≅ ΔBNC.
Using CPCT, ∠ADC = ∠BCD —————– (1)
∠BAD and ∠ADC are on the same side of transversal AD.
∠BAD + ∠ADC = 180°
∠BAD + ∠BCD = 180° [From equation(1)]
This equation proves that the sum of opposite angles is supplementary. Hence, ABCD is a cyclic quadrilateral.
9. Two circles intersect at two points, B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D and P, Q, respectively (see Fig. 10.40). Prove that ∠ ACP = ∠ QCD.
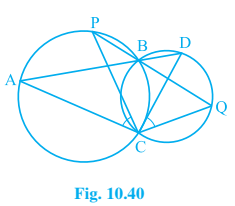
Answer – ∠ACP and ∠ABP lie in the same segment. Similarly, ∠DCQ and ∠DBQ lie in the same segment.
We know that angles in the same segment of a circle are equal.
So, we get ∠ACP = ∠ABP and ∠QCD = ∠QBD
Also, ∠QBD = ∠ABP (Vertically opposite angles)
Therefore, ∠ACP = ∠QCD.
10. If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lies on the third side.
Answer – We know that an angle in a semicircle is a right angle. By using this fact, we can show that BDC is a line that will lead to proving that the point of intersection lies on the third side.
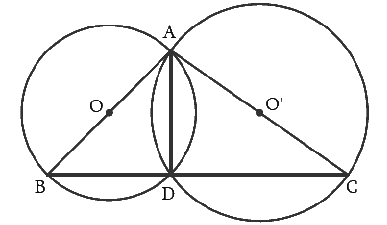
Since angle in a semicircle is a right angle, we get:
∠ADB = 90° and ∠ADC = 90°
∠ADB + ∠ADC = 90° + 90°
⇒ ∠ADB + ∠ADC = 180°
⇒ BDC is a straight line.
D lies on BC
Hence, the point of intersection of circles lies on the third side BC.
11. ABC and ADC are two right triangles with common hypotenuse AC. Prove that ∠ CAD = ∠CBD.
Answer – We know that, the sum of all angles in a triangle is 180°.
If the sum of pair of opposite angles in a quadrilateral is 180°, then it is a cyclic quadrilateral.

Consider ΔABC,
∠ABC + ∠BCA + ∠CAB = 180° (Angle sum property of a triangle)
90° + ∠BCA + ∠CAB = 180°
∠BCA + ∠CAB = 90° —————- (1)
Consider ΔADC,
∠CDA + ∠ACD + ∠DAC = 180° (Angle sum property of a triangle)
90° + ∠ACD + ∠DAC = 180°
∠ACD + ∠DAC = 90° —————- (2)
Adding Equations (1) and (2), we obtain
∠BCA + ∠CAB + ∠ACD + ∠DAC = 180°
(∠BCA +∠ACD) + (∠CAB + ∠DAC) = 180°
∠BCD + ∠DAB = 180° —————- (3)
However, it is given that
∠B + ∠D = 90° + 90° = 180° —————- (4)
From Equations (3) and (4), it can be observed that the sum of the measures of opposite angles of quadrilateral ABCD is 180°. Therefore, it is a cyclic quadrilateral.
Since it is a cylclic quadrilateral the below figure can be drawn.
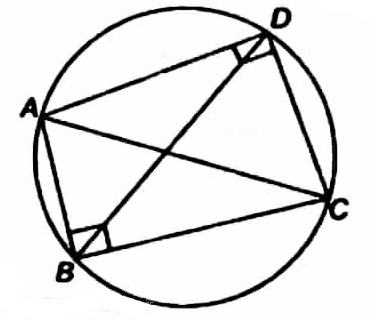
Consider chord CD. ∠CAD and ∠CBD are formed on the same segment CD.
∠CAD = ∠CBD (Angles in the same segment are equal)
12. Prove that a cyclic parallelogram is a rectangle.
Answer – The sum of either pair of opposite angles of a cyclic quadrilateral is 180°. Using this fact, we can show each angle of a cyclic parallelogram as 90°, proving the statement it is a rectangle.
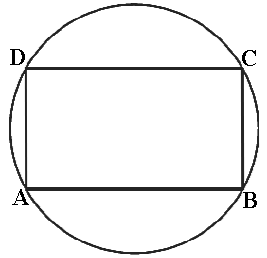
Let ABCD be the cyclic parallelogram.
We know that opposite angles of a parallelogram are equal.
∠A = ∠C and ∠B = ∠D ————— (1)
We know that the sum of either pair of opposite angles of a cyclic quadrilateral is 180°.
∠A + ∠C = 180°
∠A + ∠A = 180° (From equation (1))
2∠A = 180°
∠A = 90°
We know that if one of the interior angles of a parallelogram is 90°, all the other angles will also be equal to 90°.
Since all the angles in the parallelogram are 90°, we can say that parallelogram ABCD is a rectangle.

