NCERT Solutions Class 8 Mathematics
Chapter – 11 (Mensuration)
The NCERT Solutions in English Language for Class 8 Mathematics Chapter – 11 Mensuration Exercise 11.2 has been provided here to help the students in solving the questions from this exercise.
Chapter 11: Mensuration
- NCERT Solution Class 8 Maths Ex – 11.1
- NCERT Solution Class 8 Maths Ex – 11.3
- NCERT Solution Class 8 Maths Ex – 11.4
Exercise – 11.2
1.The shape of the top surface of a table is a trapezium. Find its area if its parallel sides are 1 m and 1.2 m and perpendicular distance between them is 0.8 m.

Solution – One parallel side of the trapezium (a) = 1 m
And second side (b) = 1.2 m and
height (h) = 0.8 m
Area of top surface of the table= × (a + b)h
= × (1 + 1.2)0.8
= × 2.2 × 0.8
= 0.88
Area of top surface of the table is 0.88 m2 .
2. The area of a trapezium is 34 cm2and the length of one of the parallel sides is 10 cm and its height is 4 cm Find the length of the other parallel side.

Solution – Let the length of the other parallel side be b.
Length of one parallel side, a = 10 cm
height, (h) = 4 cm and
Area of a trapezium is 34 cm2
Formula for, Area of trapezium = ×(a + b)h
34 = (10 + b)×4
34 = 2×(10+b)
17 = (10+b)
b = 17 – 10
b = 7
Hence another required parallel side is 7 cm.
3. Length of the fence of a trapezium shaped field ABCD is 120 m. If BC = 48 m, CD = 17 m and AD = 40 m, find the area of this field. Side AB is perpendicular to the parallel sides AD and BC.
Solution –
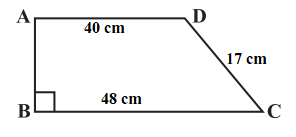
BC = 48 m,
CD = 17 m,
AD = 40 m
Perimeter = 120 m
∵ Perimeter of trapezium ABCD
= AB+BC+CD+DA
120 = AB + 48 + 17 + 40
120 = AB + 105
AB = 120 – 105
AB = 15 m
Now, Area of the field= ×(BC+AD)×AB
= ×(48 + 40) × 15
= × 88 × 15
= 660
Hence, area of the field ABCD is 660m2 .
4. The diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.
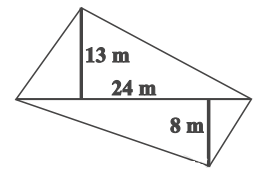
Solution –
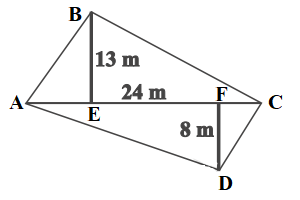
From the diagram, the area for quadrilateral will be the sum of the area of two triangles.
Area of quadrilateral ABCD = Area of ΔABC + Area of ΔADC
= × (AC × BE) +
× (AC × FD)
= × AC (BE + FD)
= × 24 m (13 m + 8 m)
= × 24 m × 21 m = 252 m²
5. The diagonals of a rhombus are 7.5 cm and 12 cm. Find its area.
Solution – Given:
d1 = 7.5 cm
d2 = 12 cm
We know that the area of a rhombus = × d1 × d2
= × 7.5 × 12 = 45
Therefore, the area of the rhombus is 45 cm2 .
6. Find the area of a rhombus whose side is 5 cm and whose altitude is 4.8 cm. If one of the diagonals is 8 cm long, find the length of the other diagonal.
Solution – Since a rhombus is also a kind of parallelogram,
The formula for Area of rhombus = Base × Altitude
Putting values, we have
Area of rhombus = 6 × 4 = 24
Area of rhombus is 24 cm2
Also, Formula for Area of rhombus = × d1 d2
After substituting the values, we get
24 = × 8 × d2
d2 = 6
Hence, the length of the other diagonal is 6 cm.
7. The floor of a building consists of 3000 tiles which are rhombus shaped and each of its diagonals are 45 cm and 30 cm in length. Find the total cost of polishing the floor, if the cost per m2is Rs. 4.
Solution – Length of one diagonal,
d1 = 45 cm
d2= 30 cm
∵ Area of one tile = d1 d2
= × 45 × 30
= 675
Area of one tile is 675 cm2
Area of 3000 tiles is
= 675 × 3000
= 2025000 cm2
= 2025000/10000
= 202.50 m2 [∵ 1m2 = 10000 cm2]
∵ Cost of polishing the floor per sq. meter = 4
Cost of polishing the floor per 202.50 sq. meter = 4 × 202.50 = 810
Hence the total cost of polishing the floor is Rs. 810.
8. Mohan wants to buy a trapezium shaped field. Its side along the river is parallel to and twice the side along the road. If the area of this field is 10500 m2 and the perpendicular distance between the two parallel sides is 100 m, find the length of the side along the river.
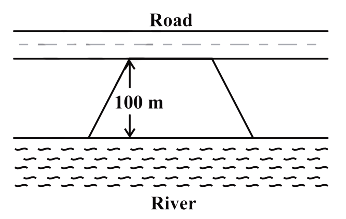
Solution – Perpendicular distance (h) = 100 m
Area of the trapezium-shaped field = 10500 m2
Let the side along the road be ‘x’ m and the side along the river = 2x m
Area of the trapezium field = × (a+b) × h
10500 = × (x + 2x) × 100
10500 = 3x × 50
150x = 10500
x = 70
which means the side along the river is 70 m
Hence, the side along the river = 2x = 2(70) = 140 m.
9. Top surface of a raised platform is in the shape of a regular octagon as shown in the figure. Find the area of the octagonal surface.
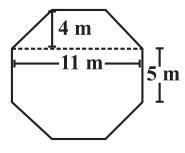
Solution – Area of rectangular surface + 2 (Area of trapeziums)
= 11 × 5 + 2 m2
= 55 + 16 × 4 m2
= 55 + 64 m2
= 119 m2
10. There is a pentagonal shaped park as shown in the figure. For finding its area Jyoti and Kavita divided it in two different ways.
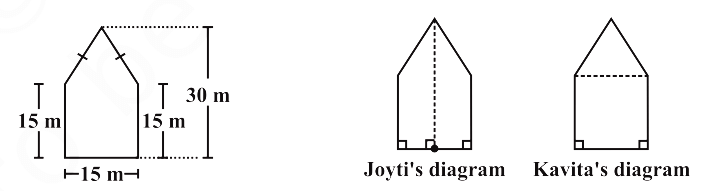
Find the area of this park using both ways. Can you suggest some other way of finding its area?
Solution – Jyoti’s diagram,
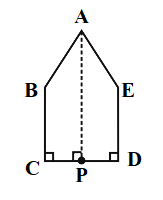
Area of pentagon = Area of trapezium ABCP + Area of trapezium AEDP
= (AP+BC)×CP +
×(ED+AP)×DP
= (30+15)×CP +
×(15+30)×DP
= × (30+15)×(CP+DP)
= ×45×CD
= ×45×15
= 337.5 m2
Area of pentagon is 337.5 m2
Kavita’s diagram
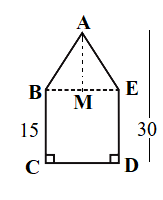
Here, a perpendicular AM is drawn to BE.
AM = 30 – 15 = 15 m
Area of pentagon = Area of triangle ABE + Area of square BCDE (from above figure)
= × 15 × 15 + (15×15)
= 112.5+225.0
= 337.5
Hence, the total area of pentagon-shaped park = 337.5 m2
11. Diagram of the adjacent picture frame has outer dimensions = 24 cm×28 cm and inner dimensions 16 cm×20 cm. Find the area of each section of the frame, if the width of each section is same.
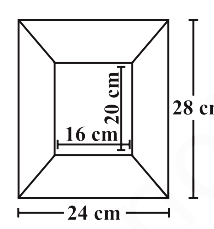
Solution –
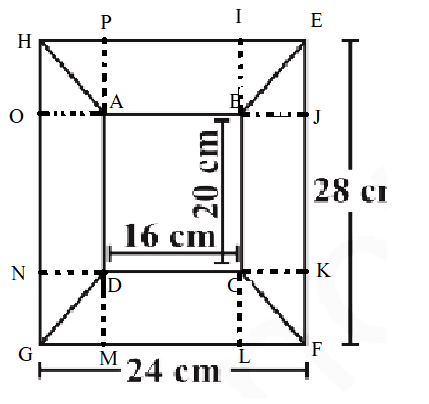
Given that the width of each section is same.
Therefore,
IB = BJ = CK = CL = DM = DN = AP = AO —————(1)
IL = IB + BC + CL
28 = IB + 20 + CL
28 − 20 = IB + CL
8 = IB + CL
But,
IB = CL [From equation (1)]
Thus, 2IB = 8
IB = 4 cm
Hence IB = BJ = CK = CL = DM = DN = AP = AO = 4 cm
We know that, ABEH, CDGF, BEFC and ADGH are all trapezium
Area of trapezium ABEH = Area of trapezium CDGF
= × (AB + HE) × IB
= (16 + 24) × 4 = 80 cm²
Area of trapezium BEFC = Area of trapezium ADGH [By symmetry]
= × (BC + EF) × BJ
= × (20 + 28) × 4 = 96 cm²
Area of rectangle ABCD = BC × DC
= 20 cm × 16 cm = 320 m²
Thus, the area of section ABEH and CDGF is 80 m², the area of section BEFC and ADGH is 96 m² and the area of section ABCD is 320 m².

