NCERT Solutions Class 6 Maths
Chapter – 3 (Playing with Numbers)
The NCERT Solutions in English Language for Class 6 Mathematics Chapter – 3 Playing with Numbers Exercise 3.6 has been provided here to help the students in solving the questions from this exercise.
Chapter 3: Playing with Numbers
- NCERT Solution Class 6 Maths Exercise – 3.1
- NCERT Solution Class 6 Maths Exercise – 3.2
- NCERT Solution Class 6 Maths Exercise – 3.3
- NCERT Solution Class 6 Maths Exercise – 3.4
- NCERT Solution Class 6 Maths Exercise – 3.5
- NCERT Solution Class 6 Maths Exercise – 3.7
Exercise – 3.6
1. Find the HCF of the following numbers :
(a) 18, 48
(b) 30, 42
(c) 18, 60
(d) 27, 63
(e) 36, 84
(f) 34, 102
(g) 70, 105, 175
(h) 91, 112, 49
(i) 18, 54, 81
(j) 12, 45, 75
Solutions:
(a) 18, 48
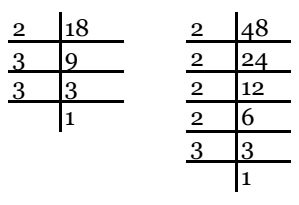
18 = 2 × 3 × 3
48 = 2 × 2 × 2 × 2 × 3
HCF = 2 × 3 = 6
Therefore the HCF of 18, 48 is 6
(b) 30, 42
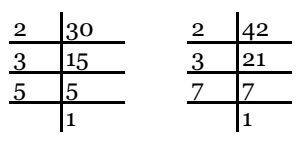
30 = 2 × 3 × 5
42 = 2 × 3 × 7
HCF = 2 × 3 = 6
Therefore the HCF of 30, 42 is 6
(c) 18, 60
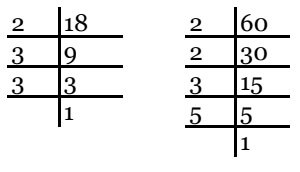
18 = 2 × 3 × 3
60 = 2 × 2 × 3 × 5
HCF = 2 × 3 = 6
Therefore the HCF of 18, 60 is 6
(d) 27, 63
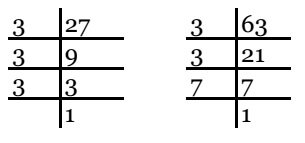
27 = 3 × 3 × 3
63 = 3 × 3 × 7
HCF = 3 × 3 = 9
Therefore the HCF of 27, 63 is 9
(e) 36, 84
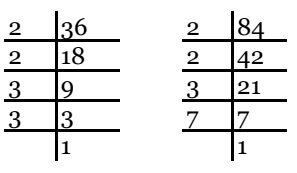
36 = 2 × 2 × 3 × 3
84 = 2 × 2 × 3 × 7
HCF = 2 × 2 × 3 = 12
Therefore the HCF of 36, 84 is 12
(f) 34, 102
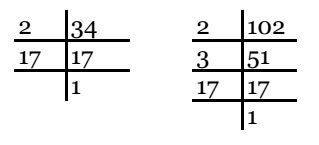
34 = 2 × 17
102 = 2 × 3 × 17
HCF = 2 × 17 = 34
Therefore the HCF of 34, 102 is 34
(g) 70, 105, 175
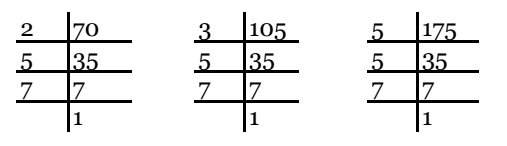
70 = 2 × 5 × 7
105 = 3 × 5 × 7
175 = 5 × 5 × 7
HCF = 5 × 7 = 35
Therefore the HCF of 70, 105, 175 is 35
(h) 91, 112, 49

91 = 7 × 13
112 = 2 × 2 × 2 × 2 × 7
49 = 7 × 7
HCF = 7
Therefore the HCF of 91, 112, 49 is 7
(i) 18, 54, 81

18 = 2 × 3 × 3
54 = 2 × 3 × 3 × 3
81 = 3 × 3 × 3 × 3
HCF = 3 × 3 = 9
Therefore the HCF of 18, 54, 81 is 9
(j) 12, 45, 75
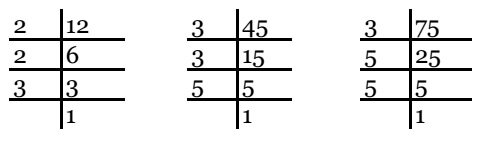
12 = 2 × 2 × 3
45 = 3 × 3 × 5
75 = 3 × 5 × 5
HCF = 3
Therefore the HCF of 12, 45, 75 is 3
2. What is the HCF of two consecutive
(a) numbers?
(b) even numbers?
(c) odd numbers?
Solutions:
(a) The HCF of two consecutive numbers is 1
Example:
The HCF of 2 and 3 is 1
(b) The HCF of two consecutive even numbers is 2
Example:
The HCF of 2 and 4 is 2
(c) The HCF of two consecutive odd numbers is 1
Example:
The HCF of 3 and 5 is 1
3. HCF of co-prime numbers 4 and 15 was found as follows by factorisation:
4 = 2 × 2 and 15 = 3 × 5
since there is no common prime factor, so HCF of 4 and 15 is 0. Is the answer correct? If not, what is the correct HCF?
Solutions:
No. The answer is not correct. The correct answer is 1.

