NCERT Solutions Class 10 Maths
Chapter – 3 (Pair of Linear Equations in Two Variables)
The NCERT Solutions in English Language for Class 10 Mathematics Chapter – 3 Pair of Linear Equations in Two Variables Exercise 3.1 has been provided here to help the students in solving the questions from this exercise.
Chapter : 3 Pair of Linear Equations in Two Variables
- NCERT Class 10 Maths Solution Ex – 3.2
- NCERT Class 10 Maths Solution Ex – 3.3
- NCERT Class 10 Maths Solution Ex – 3.4
- NCERT Class 10 Maths Solution Ex – 3.5
- NCERT Class 10 Maths Solution Ex – 3.6
- NCERT Class 10 Maths Solution Ex – 3.7
Exercise – 3.1
1. Aftab tells his daughter, “Seven years ago, I was seven times as old as you were then. Also, three years from now, I shall be three times as old as you will be.” (Isn’t this interesting?) Represent this situation algebraically and graphically.
Solutions –
Let the present age of Aftab be ‘x’.
And, the present age of his daughter be ‘y’.
Now, we can write, seven years ago,
Age of Aftab = x – 7
Age of his daughter = y – 7
According to the question,
x − 7 = 7(y − 7)
x − 7 = 7y − 49
x − 7y = −42 —————- (i)
Also, three years from now or after three years,
Age of Aftab will become = x + 3.
Age of his daughter will become = y + 3
According to the situation given,
x + 3 = 3(y + 3)
x + 3 = 3y + 9
x − 3y = 6 —————– (ii)
Subtracting equation (i) from equation (ii) we have
(x − 3y) − (x − 7y) = 6 − (−42)
−3y + 7y = 6 + 42
4y = 48
y = 12
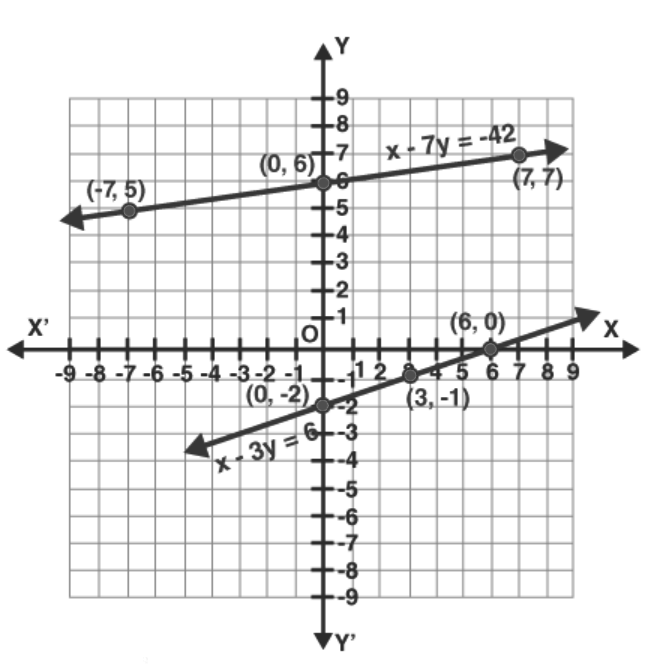
The algebraic equation is represented by
x − 7y = −42
x − 3y = 6
For, x − 7y = −42
x = −42+7y
The solution table is
| x | -7 | 0 | 7 |
| y | 5 | 6 | 7 |
For, x − 3y = 6
x = 6 + 3y
The solution table is
| x | 6 | 3 | 0 |
| y | 0 | -1 | -2 |
The graphical representation is:
2. The coach of a cricket team buys 3 bats and 6 balls for Rs.3900. Later, she buys another bat and 3 more balls of the same kind for Rs.1300. Represent this situation algebraically and geometrically.
Solutions – Let us assume that the cost of a bat be Rs ‘x’
And, the cost of a ball be Rs ‘y’
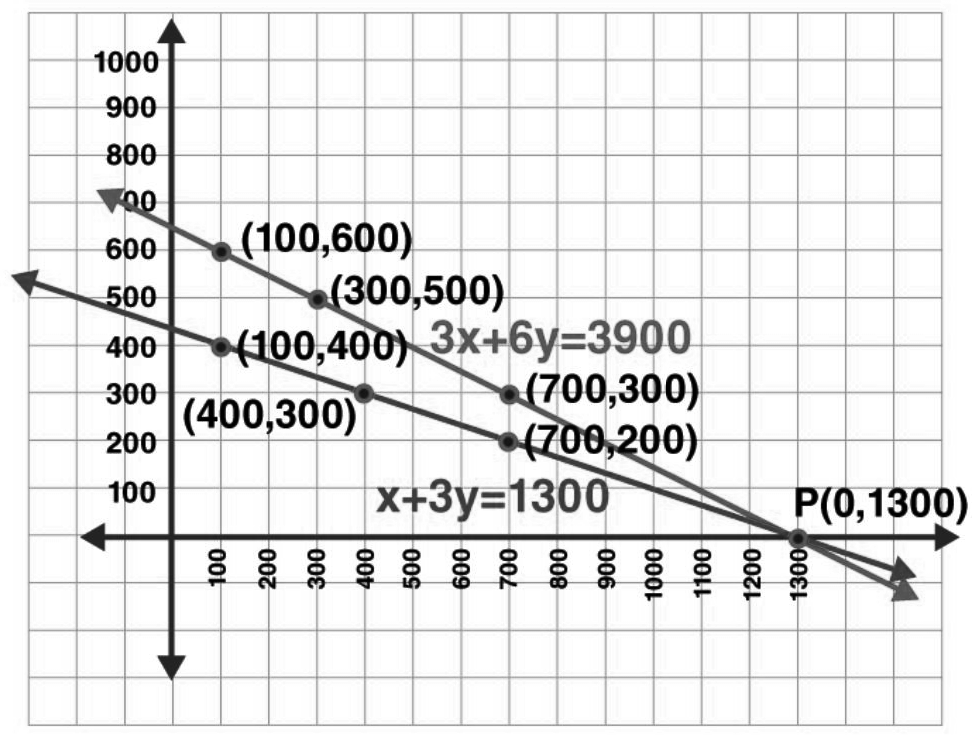
According to the question, the algebraic representation is
3x + 6y = 3900 ————– (i)
x + 3y = 1300 ————– (ii)
For 3x + 6y = 3900
x + 2y = 1300
x = 1300 – 2y
The solution table is
| y | 500 | 600 | 300 |
| x | 300 | 100 | 700 |
For, x + 3y = 1300
x = 1300 – 3y
The solution table is
| y | 300 | 400 | 200 |
| x | 400 | 100 | 700 |
The graphical representation is as follows.
3. The cost of 2 kg of apples and 1kg of grapes on a day was found to be Rs.160. After a month, the cost of 4 kg of apples and 2 kg of grapes is Rs.300. Represent the situation algebraically and geometrically.
Solutions – Let the cost of 1 kg of apples be Rs. ‘x’
And, cost of 1 kg of grapes be Rs. ‘y’
According to the question, the algebraic representation is
2x + y = 160 ————- (i)
4x + 2y = 300 ————- (ii)
For, 2x + y = 160
y = 160 − 2x
the solution table is;
| y | 60 | 40 | 20 |
| x | 50 | 60 | 70 |
For 4x + 2y = 300
y = (300 – 4x)/2,
y = 150 – 2x
the solution table is;
| y | 10 | -10 | 0 |
| x | 70 | 80 | 75 |
The graphical representation is as follows;


