NCERT Solutions Class 6 Maths
Chapter – 13 (Symmetry)
The NCERT Solutions in English Language for Class 6 Mathematics Chapter – 13 Symmetry Exercise 13.2 has been provided here to help the students in solving the questions from this exercise.
Chapter 13: Symmetry
Exercise – 13.2
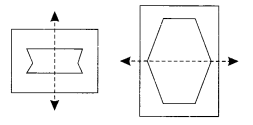
1. Find the number of lines of symmetry for each of the following shapes :
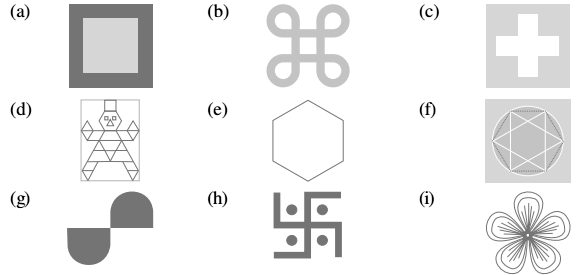
Solutions:
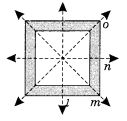
(a) Here, there are 4 symmetric lines l, m, n and o.
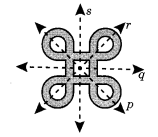
(b) In this figure, there are 4 symmetric lines p, q, r and s.
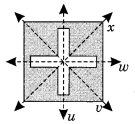
(c) In this shape, u, v , w and x are 4 lines of symmetry.
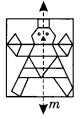
(d) In this shape only 1, m is the line of symmetry.
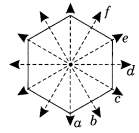
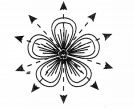
(e) Here, a, b, c, d, e and fare 6 lines of symmetry.
(f) In this figure l, m, n, o, p and q are 6 lines of symmetry.

(g) This figure has no lines of symmetry.
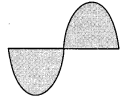
(h) This figure has no lines of symmetry.
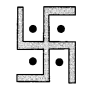
(i) This figure has 5 lines of symmetry.
2. Copy the triangle in each of the following figures on squared paper. In each case, draw the line(s) of symmetry, if any and identify the type of triangle. (Some of you may like to trace the figures and try paper-folding first!)
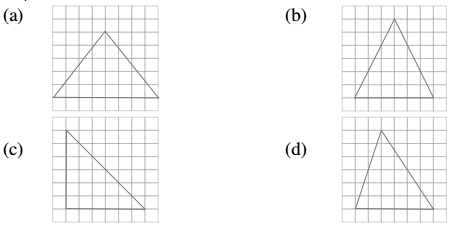
Solutions:
(a) The given triangle is an isosceles triangle. Here it will be only 1 line of symmetry.
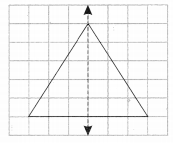
(b) The given triangle is an isosceles triangle. Here it will be only 1 line of symmetry.

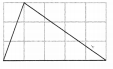
(c) The given triangle is a right angled triangle. Here it will be only 1 line of symmetry.
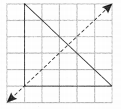
(d) The given triangle is a scalene triangle. Here it will be no line of symmetry.
3. Complete the following table.
| Shape | Rough figure | Number of lines of symmetry |
| Equilateral triangle |  |
3 |
| Square | ||
| Rectangle | ||
| Isosceles triangle | ||
| Rhombus | ||
| Circle |
Solutions:
The given table is completed as follows:
| Shape | Rough figure | Number of lines of symmetry |
| Equilateral triangle |  |
3 |
| Square | 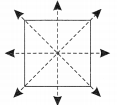 |
4 |
| Rectangle | 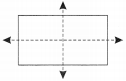 |
2 |
| Isosceles triangle | 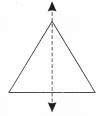 |
1 |
| Rhombus |  |
2 |
| Circle | 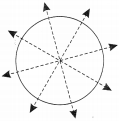 |
Infinite |
4. Can you draw a triangle which has
(a) exactly one line of symmetry?
(b) exactly two lines of symmetry?
(c) exactly three lines of symmetry?
(d) no lines of symmetry?
Sketch a rough figure in each case.
Solutions:
(a) Yes, we can draw an isosceles triangle which has only 1 line of symmetry.
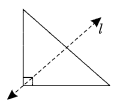
(b) No, we cannot draw any triangle with two symmetric lines.
(c) Yes, equilateral triangle has three lines of symmetry.
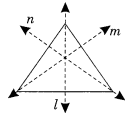
(d) Yes, Scalene triangle has no lines of symmetry.
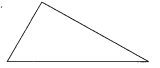
5. On a squared paper, sketch the following:
(a) A triangle with a horizontal line of symmetry but no vertical line of symmetry.
(b) A quadrilateral with both horizontal and vertical lines of symmetry.
(c) A quadrilateral with a horizontal line of symmetry but no vertical line of symmetry.
(d) A hexagon with exactly two lines of symmetry.
(e) A hexagon with six lines of symmetry.
Solutions:
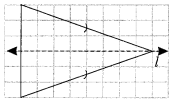
(a) A triangle with a horizontal line of symmetry but no vertical line of symmetry can be drawn as follows.
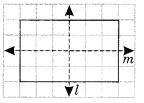
(b) A quadrilateral with both horizontal and vertical lines of symmetry can be drawn as follows.
(c) Trapezium (quadrilateral) shows the horizontal but no vertical line of symmetry can be drawn as follows.

(d) A hexagon with exactly two lines of symmetry may be drawn as follows.
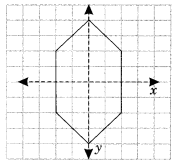
(e) A hexagon with 6 lines of symmetry may be drawn as follows.

6. Trace each figure and draw the lines of symmetry, if any:
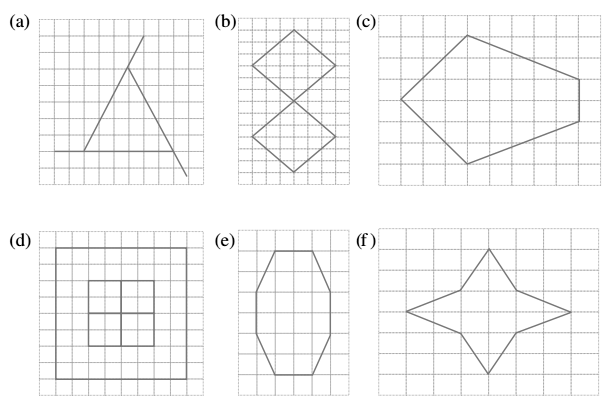
Solutions:
(a) The given figure has no line of symmetry as it is not symmetrical.
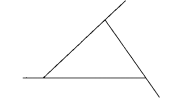
(b) The given figure has 2 lines of symmetry.
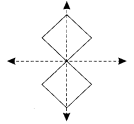
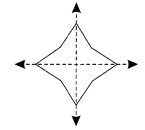
(c) The given figure has 4 lines of symmetry.
(d) The given figure has 2 lines of symmetry.
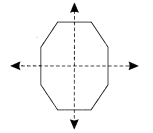
(e) This figure has only 1 horizontal line of symmetry.
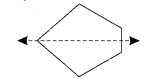
(f) The given figure has 2lines of symmetry.
7. Consider the letters of English alphabets, A to Z. List among them the letters which have
(a) vertical lines of symmetry (like A)
(b) horizontal lines of symmetry (like B)
(c) no lines of symmetry (like Q)
Solutions:
(a) Vertical lines of symmetry are A, H, I, M, O, T, U, V, W, X, Y.
(b) Horizontal lines of symmetry are B, C, D, E, H, I, K, O, X.
(c) No lines of symmetry are F, G, J, L, N, P, Q, R, S, Z.
8. Given here are figures of a few folded sheets and designs drawn about the fold. In each case, draw a rough diagram of the complete figure that would be seen when the design is cut off.
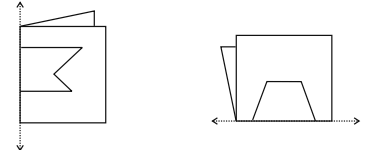
Solutions:
We may draw a diagram of complete figure as follows